Chapter VII THERMAL RADIATION AND ITS EFFECTS
RADIATION FROM THE FIREBALL
GENERAL CHARACTERISTICS OF THERMAL RADIATION
7.01 One of the important differences between a nuclear and a conventional high-explosive weapon is the large proportion of the energy of a nuclear explosion which is released in the form of thermal (or heat) radiation. Because of the enormous amount of energy liberated per unit mass in a nuclear weapon, very high temperatures are attained. These are estimated to be several tens of million degrees, compared with a few thousand degrees in the case of a conventional explosion. As a consequence of these high temperatures, about 70 to 80 percent of the total energy (excluding the energy of the residual radiation) is released in the form of electromagnetic radiation of short wavelength. Initially, the (primary) thermal radiations are mainly in the soft X-ray region of the spectrum but, for nuclear explosions below about 50 miles, the X rays are absorbed in air in the general vicinity of the burst, thereby heating it to high temperatures. Most of the remaining 20 to 30 percent of the energy is initially in the form of kinetic energy of the weapon debris. This kinetic energy is also absorbed by the air at a slightly later time (§ 2.109) and serves to further heat the air. The heated air, which constitutes the fireball, in turn radiates in a spectral region roughly similar to that of sunlight near the earth’s surface. It is the radiation (ultra violet, visible, and infrared) from the fireball, traveling with the velocity of light, which constitutes the thermal radiation at distances from the explosion. The time elapsing, therefore, between the emission of this (secondary) thermal radiation from the fireball and its arrival at a target miles away, is quite insignificant.
7.02 It is desirable to state specifically what is meant by the term “thermal radiation” as it is used in the present chapter. Actually, all the energy released by a nuclear detonation, including the residual radiation from the weapon debris, is ultimately degraded to thermal energy, i.e., heat. But only part of it is regarded as constituting the thermal radiation of interest which can cause fire damage and personal injury at or near the earth’s surface. Some of the thermal radiations emitted by the fire ball in the very early stages, particularly in the ultraviolet region, are selectively absorbed by various atomic and molecular species in the heated air, which slowly re-emits this energy in a degraded, i.e., longer wavelength, form. The delay in reaching the target, and the slower rate at which they are delivered, lowers the damaging effectiveness of these radiations. Consequently they are not considered as a part of the thermal radiation for present purposes. It is convenient, therefore, to define the effective (or prompt) thermal radiation as that emitted from the heated air of the fireball within the first minute (or less) following the explosion.
7.03 For an air burst at altitudes below about 100,000 feet (roughly 19 miles), the thermal radiation is emitted from the fireball in two pulses, as described in Chapter II. The first, which is quite short, carries roughly 1 percent of the total radiant energy (§ 2.39); the second pulse is the more significant and is of longer duration. The total length of the effective thermal pulse increases with the energy yield of the explosion. Thus the duration of the effective pulse from a 1-kiloton airburst is about 0.4 second, whereas from a 10-megaton explosion it is more than 20 seconds. With increasing altitude the character of the thermal radiation pulse changes (§ 2.130 et seq.). At altitudes above about 100,000 feet, there is only a single thermal pulse and its effective duration, which depends on the height of burst and the energy yield of the explosion, is of the order of a second or less for weapons in the megaton range. For explosions above about 270,000 feet (51 miles), the pulse length is somewhat longer.
7.04 In an ordinary air burst, i.e., at altitudes up to some 100,000 feet, roughly 35 to 45 percent of the total energy yield of the explosion is emitted as effective thermal radiation. The actual fraction of the energy that appears as such radiation depends on the height of burst and the total yield, as well as on the weapon characteristics; estimates of this fraction for various yields and burst altitudes will be given later (Table 7.88). For simplicity, however, it is often assumed that 35 percent of the total energy yield of an air burst is emitted as thermal radiation energy. This means that for every 1 kiloton TNT equivalent of energy release, about 0.35 kiloton, i.e., 3.5 × 1011 calories or about 410,000 kilowatt-hours, is in the form of thermal radiation. The proportion of this energy that reaches the surface depends on the distance from the burst point and on the state of the atmosphere.
7.05 A nuclear air burst can cause considerable blast damage; however, thermal radiation can result in serious additional damage by igniting combustible materials, e.g., finely _divided or thin fuels such as dried leaves and newspapers. Thus, fires may be started in buildings and forests and may spread rapidly to considerable distances. In addition, thermal radiation is capable of causing skin burns and eye injuries to exposed persons at distances at which thin fuels are not ignited. Thermal radiation can, in fact, be an important cause of injuries to people from both direct exposure and as the result of fires, even at greater distances than other weapons effects.
ATTENUATION OF THERMAL RADIATION
7.06 The extent of injury or damage caused by thermal radiation or the chance of igniting combustible material depends to a large extent upon the amount of thermal radiation energy received by a unit area of skin, fabric, or other exposed material within a short interval of time. The thermal energy falling upon a given area from a specified explosion will be less the farther from the explosion, for two reasons: (1) the spread of the radiation over an ever increasing area as it travels away from the fireball, and (2) attenuation of the radiation in its passage through the air. These factors will be considered in turn.
7.07 If the radiation is distributed evenly in all directions, then at a distance from the explosion the same amount of energy will fall upon each unit area of the surface of a sphere of radius . The total area of this sphere is , and if is the thermal radiation energy produced in the explosion, the energy received per unit area at a distance would be , provided there were no attenuation by the atmosphere. Obviously, this quantity varies inversely as the square of the distance from the explosion. At 2 miles, from a given explosion, for example, the thermal energy received per unit area would be one-fourth of that received at half the distance, i.e., at 1 mile, from the same explosion.
7.08 In order to estimate the amount of thermal energy actually reaching the unit area, allowance must also be made for the attenuation of the radiation by the atmosphere. This attenuation is due to two main causes, namely, absorption and scattering. Atoms and molecules present in the air are capable of absorbing, and thus re moving, certain portions of the thermal radiation. The absorption is most effective for the shorter wavelength (or ultraviolet) rays. In this connection, oxygen molecules, as well as ozone, nitrogen dioxide, and nitrous acid formed from the gases in the atmosphere (§ 2.123), play an important part.
7.09 Because of absorption, the thermal radiation, particularly that in the ultraviolet region, decreases markedly with increasing distance from the explosion. Some of the absorbed radiation is subsequently reradiated, but the emission occurs with equal probability in all directions, so that the quantity proceeding in the direction of a given target is substantially reduced. Consequently, at those distances where persons exposed to thermal radiation could survive the blast and initial nuclear radiation effects, the proportion of ultraviolet radiation is quite small. However, the ultraviolet is more effective in causing biological injury than visible and infrared rays, so that even the small amount present could, under some conditions, be important.
7.10 Attenuation as a result of scattering, i.e., by the random diversion of rays from their original paths, occurs with radiations of all wavelengths. Scattering can be caused by molecules, such as oxygen and nitrogen, present in the air. This is, however, not as important as scattering resulting from the reflection and diffraction (or bending) of light rays by particles, e.g., of dust, smoke, or fog, in the atmosphere. The diversion of the radiation as a result of scattering interactions leads to a somewhat diffuse, rather than a direct, transmission of the thermal radiation.
EFFECT OF ATMOSPHERIC CONDITIONS
7.11 The decrease in energy of thermal radiation due to scattering by particles in the air depends upon the atmospheric conditions, such as the concentration and size of the particles, and also upon the wavelength of the radiation. This means that radiations of different wavelengths, namely, ultraviolet, visible, and infrared, will suffer energy attenuation to different extents. For most practical purposes, however, it is more convenient and reasonably satisfactory, although less precise, to postulate a mean attenuation averaged over all the wavelengths present in the thermal radiation.
7.12 The extent to which the atmosphere attenuates thermal energy and limits visibility depends largely on the scattering of radiation. Therefore, the state of the atmosphere as far as scattering is concerned can be represented by what is known as the daylight “visibility range” or, in brief, as the “visibility.” This is defined as the horizontal distance at which a large dark object on the horizon has just enough contrast with the surrounding sky to be discernible in daylight. The international code for correlating the visibility with the condition of the atmosphere is given in Table 7.12.
| Visibility | ||
|---|---|---|
| Atmospheric Condition | Kilometers | Miles |
| Exceptionally clear | 280 | 170 |
| Very clear | 50 | 31 |
| Clear | 20 | 12 |
| Light haze | 10 | 6 |
| Haze | 4 | 2.5 |
| Thin fog | 2 | 1.2 |
| Light to thick fog | 1 or less | 0.6 or less |
7.13 At first thought, it would be expected that the decrease of thermal radiation energy with increasing distance from the explosion would be greater when the visibility is low than when it is high. But, for the reason given below, it has been found that, at distances less than about half the visibility, the degree of attenuation of the thermal radiation is relatively insensitive to atmospheric conditions if at least moderately clear (10 miles or more) visibility prevails. At greater distances, however, a larger proportion of the radiant energy is indeed lost as the atmospheric visibility decreases. As a rough approximation, the amount of thermal energy received at a given distance from a nuclear explosion may be assumed to be independent of the visibility. This leads to overestimates at distances greater than about half the visibility range, but from the standpoint of protection from thermal radiation such estimates would be preferable to those which err in being too low.
7.14 The thermal radiation received at a given distance from a nuclear explosion is made up of both directly transmitted (unscattered) and scattered radiations. If the air is clear, and there are very few suspended particles, the extent of scattering is small, and the radiation received is essentially only that which has been transmitted from the exploding weapon without scattering. If the air contains a moderately large number of particles, the amount of radiation transmitted directly will be less than in a clear atmosphere. But this decrease is largely compensated by an increase in the scattered radiation reaching the object (or area) under consideration. Multiple scattering, i.e., subsequent scattering of already scattered radiation, which is very probable when the concentration of particles is high, will result in the arrival of radiation at the target from many directions. An appreciable amount of thermal radiation will thus reach the given area in directly, in addition to that transmitted directly. It is because of the partial compensation due to multiple scattering that the total amount of energy from a nuclear explosion falling upon a unit area at a given distance may not be too greatly dependent upon the visibility range, within certain limits.
7.15 Under atmospheric conditions of rain, fog, or dense industrial haze, absorption due to the increase in water vapor and carbon dioxide content of the air will play a predominant role in the attenuation of thermal radiation. The loss in the directly transmitted radiation, from scattering and absorption, cannot then be compensated by multiple scattering. Hence, less radiant energy is received at a specified distance from the explosion than for clear visibility conditions.
EFFECT OF SMOKE, FOG, AND CLOUDS
7.16 In the event of an air burst occurring above a layer of dense cloud, smoke, or fog, an appreciable portion of the thermal radiation will be scattered upward from the top of the layer. This scattered radiation may be regarded as lost, as far as a point on the ground is concerned. In addition, most of the radiation which penetrates the layer will be scattered, and very little will reach the given point by direct transmission. These two effects will result in a substantial decrease in the amount of thermal energy reaching a ground target covered by fog or smoke, from a nuclear explosion above the layer.
7.17 It is important to understand that the decrease in thermal radiation by fog and smoke will be realized only if the burst point is above or, to a lesser extent, within the fog (or similar) layer. If the explosion should occur in moderately clear air beneath a layer of cloud or fog, some of the radiation which would normally proceed outward into space will be scattered back to earth. As a result, there may be some cases in which the thermal energy received will actually be greater than for the same atmospheric transmission conditions without a cloud or fog cover. (A layer of snow on the ground will have much the same effect as a cloud layer above the burst (§§ 7.43, 7.100)).
EFFECT OF SHIELDING
7.18 Unless it is scattered, thermal radiation from a nuclear explosion, like ordinary light, travels in straight lines from the fireball. Any solid, opaque material, e.g., a wall,. a hill, or a tree, between a given object and the fireball will act as a shield and provide protection from thermal radiation. Some instances of such shielding, many of which were observed after the nuclear explosions in Japan, will be described later. Transparent materials, on the other hand, such as glass or plastics, allow thermal radiation to pass through only slightly attenuated.
7.19 A shield which merely intervenes between a given target and the fireball but does not surround the target, may not be entirely effective under hazy atmospheric conditions. A large proportion of the thermal radiation received, especially at considerable distances from the explosion, has undergone multiple scattering and will arrive from all directions, not merely that from the point of burst. This situation should be borne in mind in connection with the problem of thermal radiation shielding.
TYPE OF BURST
7.20 The foregoing discussion has referred in particular to thermal radiation from a nuclear air burst. For other types of burst the general effects are the same, although they differ in degree. For a surface burst, in which the fireball actually touches the earth or water, the proportion of the explosion energy appearing at a distance as thermal radiation will be less than for an air burst. Some energy is utilized in melting or evaporating surface material, but this is relatively small (about 1 or 2 percent) and has a minor effect on the thermal radiation emitted. As far as the energy received at a distance from the explosion is concerned, other factors are more significant. First, there will be a certain amount of shielding due to terrain irregularities and, second, some absorption of the radiation will occur in the low layer of dust or water vapor produced near the burst point in the early stages of the explosion. In addition, most of the thermal radiation reaching a given target on the ground will have traveled through the air near the earth’s surface. In this part of the atmosphere there is considerable absorption by molecules of water vapor and of carbon dioxide and the extent of scattering by various particles is greater than at higher altitudes. Consequently, in a surface burst, the amount of thermal energy reaching a target at a specified distance from the explosion may be from half to three-fourths of that from an air burst of the same total energy yield. However, when viewed from above, e.g., from an aircraft, surface explosions exhibit the same thermal characteristics as air bursts.
7.21 In subsurface bursts, either in the earth or under water, nearly all the thermal radiation is absorbed, provided there is no appreciable penetration of the surface by the fireball. The thermal energy is then used up in heating and melting the soil or vaporizing the water, as the case may be. Normal thermal radiation effects, such as accompany an air burst, are thus absent.
7.22 When nuclear explosions occur at high altitudes, i.e., somewhat above 100,000 feet, the primary thermal X rays from the extremely hot weapon residues are absorbed in a large volume (and mass) of air because of the low density, as explained in § 2.131 et seq. Consequently, the fireball temperatures are lower than for an air burst at lower altitude (§ 7.81), with the result that, although about half of the absorbed energy is emitted as thermal radiation in less than a second, the remainder of the thermal energy is radiated so slowly that it can be ignored as a significant effect.
THERMAL RADIATION EFFECTS
ABSORPTION OF THERMAL RADIATION
7.23 The amount of thermal energy falling upon a unit area exposed to a nuclear explosion depends upon the total energy yield, the height of burst, the distance from the explosion, and, to some extent, the atmospheric conditions. The thermal radiation leaving the fireball covers a wide range of wavelengths, from the short ultraviolet, through the visible, to the infrared region. Much of the ultraviolet radiation is absorbed or scattered in its passage through the atmosphere with the result that at a target near the earth’s surface less ultraviolet radiation is received than might be expected from the temperature of the fireball.1
7.24 When thermal radiation falls upon any material or object, part may be reflected, part will be absorbed, and the remainder, if any, will pass through and ultimately fall upon other materials. It is the radiation absorbed by a particular material that produces heat and so determines the damage suffered by that material. The extent or fraction of the incident radiation that is absorbed de pends upon the nature and color of the material or object. Highly reflecting and transparent substances do not absorb much of the thermal radiation and so they are relatively resistant to its effects. A thin material will often transmit a large proportion of the radiation falling upon it and thus escape serious damage. A black fabric will absorb a much larger proportion of the incident thermal radiation than will the same fabric when white in color. The former will thus be more affected than the latter. A light colored material will then not char as readily as a dark piece of the same material.
7.25 Essentially all of the thermal radiation absorbed serves to raise the temperature of the absorbing material and it is the high temperature attained which causes injury or damage, or even ignition of combustible materials. An important point about the thermal radiation from a nuclear explosion is not only that the amount of energy is considerable, but also that it is emitted in a very short time. This means that the intensity of the radiation, i.e., the rate at which it is incident upon a particular surface, is very high. Because of this high intensity, the heat accompanying the absorption of the thermal radiation is produced with great rapidity.
7.26 Since only a small proportion of the heat is dissipated by conduction in the short time during which the radiation falls upon the material-except perhaps in good heat conductors such as metals-the absorbed energy is largely confined to a shallow depth of the material. Consequently, very high temperatures are attained at the surface. It has been estimated, for example, that in the nuclear explosions in Japan (§ 2.24), solid materials on the ground immediately below the burst probably attained surface temperatures of 3,000 to 4,000°C (5,400 to 7,200°F). It is true that the temperatures fell off rapidly with increasing distance from the explosion, but there is some evidence that they reached 1,800°C (3,270°F) at 3,200 feet (0.61 mile) away (§ 7.47).
7.27 The most important physical effects of the high temperatures resulting from the absorption of thermal radiation are burning of the skin, and scorching, charring, and possibly ignition of combustible organic substances, e.g., wood, fabrics, and paper (Fig. 7.27). Thin or porous materials, such as lightweight fabrics, newspaper, dried grass and leaves, and dry rotted wood, may flame when exposed to thermal radiation. On the other hand, thick organic materials, for example, wood (more than ½ inch thick), plastics, and heavy fabrics, char but do not burn. Dense smoke, and even jets of flame, may be emitted, but the material does not sustain ignition. If the material is light colored and blackens readily by charring in the initial stages of exposure to thermal radiation, it will absorb the subsequent thermal radiation more readily. However, smoke formed in the early stages will partially shield the underlying material from subsequent radiation.

7.28 This behavior is illustrated in the photographs taken of one of the wood-frame houses exposed in the 1953 Nevada tests. As mentioned in § 5.55, the houses were given a white exterior finish in order to reflect the thermal radiation and minimize the chances of fire. Virtually at the instant of the burst, the house front became covered with a thick black smoke, as shown in Fig. 7.28a. There was, however, no sign of flame. Very shortly thereafter, but before the arrival of the blast wave, i.e., within less than 2 seconds from the explosion, the smoke ceased, as is apparent from Fig. 7.28b. Ignition of the wood did not occur.

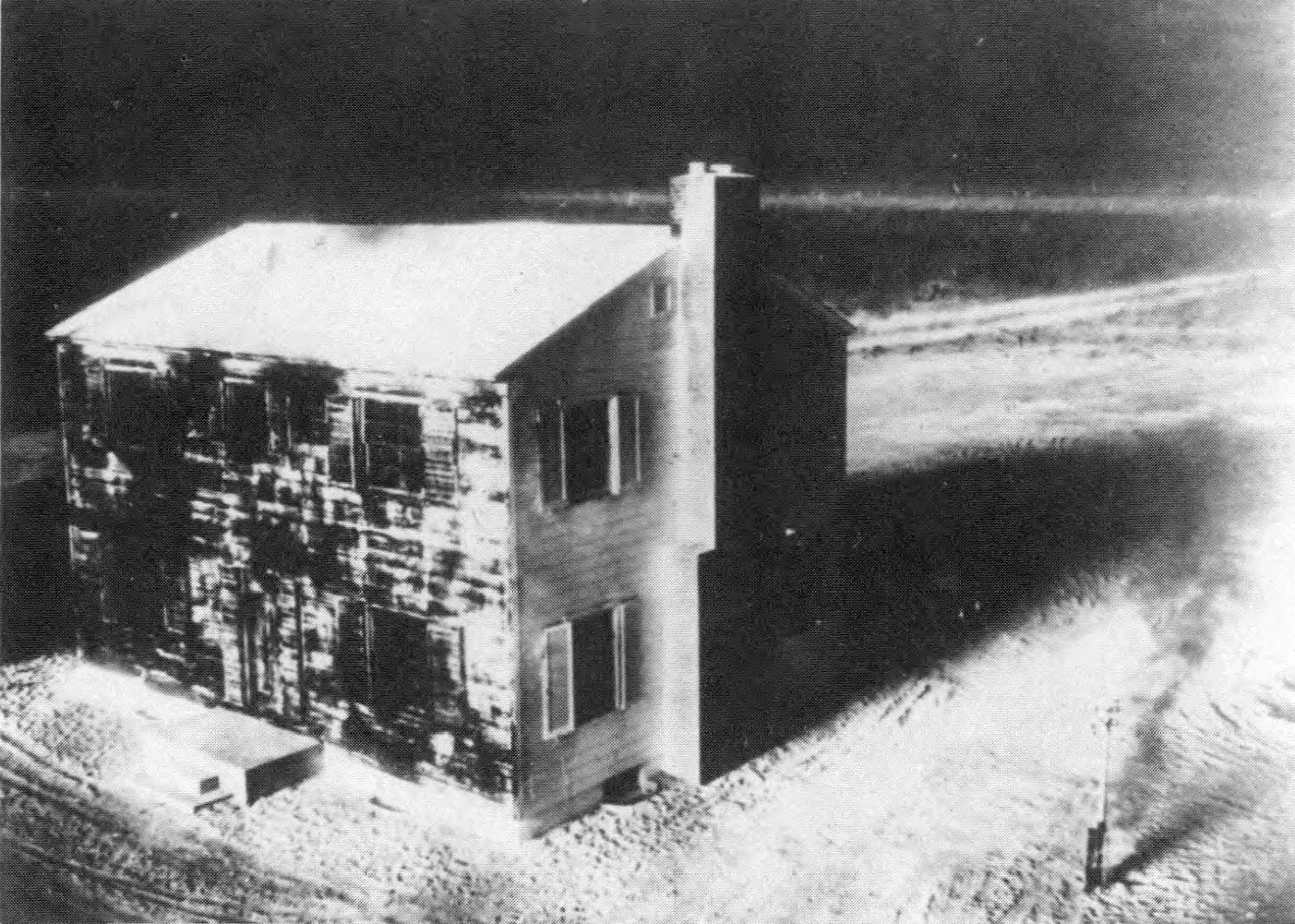
7.29 The ignition of materials by thermal radiation depends upon a number of factors, the two most important, apart from the nature of the material itself, being the thickness and the moisture content. A thin piece of a given material, for example, will ignite more easily than a thick one, and a dry sample will be more readily damaged than one that is damp.
7.30 An important consideration in connection with charring and ignition of various materials and with the production of skin burns by thermal radiation is the rate at which the thermal energy is delivered. For a given total amount of thermal energy received by each unit area of exposed material, the damage will be greater if the energy is delivered rapidly than if it were delivered slowly. This means that, in order to produce the same thermal effect in a given material, the total amount of thermal energy (per unit area) received must be larger for a nuclear explosion of high yield than for one of the lower yield, because a given amount of energy is delivered over a longer period of time, i.e., more slowly, in the former case.
7.31 There is evidence that for thermal radiation pulses of very short duration, such as might arise from air bursts of low-yield weapons or from explosions of large yield at high altitudes, this trend is reversed. In other words, a given amount of energy may be less effective if delivered in a very short pulse, e.g., a fraction of second, than in one of moderate duration, e.g., one or two seconds. In some experiments in which certain materials were exposed to short pulses of thermal radiation, it was observed that the surfaces were rapidly degraded and vaporized. It appeared as if the surface had been “exploded” off the material, leaving the remainder with very little sign of damage. The thermal energy incident upon the material was apparently dissipated in the kinetic energy of the “exploding” surface molecules before the radiation could penetrate into the depth of the material.
THERMAL RADIATION EFFECTS ON SKIN AND EYES
7.32 One of the serious consequences of the thermal radiation from a nuclear explosion is the production of “flash burns” resulting from the absorption of radiant energy by the skin of exposed individuals. In addition, because of the focusing action of the lens of the eye, thermal radiation can cause permanent damage to the eyes of persons who happen to be looking directly at the burst; however, such direct viewing will be fortuitous and rare. What is expected to be a more frequent occurrence, and therefore much more important to defensive action, is the temporary loss of visual acuity (flash blindness or dazzle) resulting from the extreme brightness, particularly at night when the eyes have been adapted to the dark. This may be experienced no matter what the direction in which the individual is facing. The various effects of thermal radiation on human beings will be considered more fully in Chapter XII.
THERMAL RADIATION DAMAGE TO FABRICS, WOOD, AND PLASTICS
7.33 Mention has already been made of the damage caused to fabrics by the high surface temperatures accompanying the absorption of thermal radiation. Natural fibers, e.g., cotton and wool, and some synthetic materials, e.g., rayon, will scorch, char, and perhaps burn; nylon, on the other hand, melts when heated to a sufficient extent. The heat energy required to produce a particular change in a fabric depends on a variety of circumstances. The following generalizations, however, appear to hold in most instances.
7.34 Dark-colored fabrics absorb the radiation, and hence suffer damage more readily than do the same fabrics if light in color. Even in this connection there are variations according to the method of dyeing and the particular fiber involved. Wool is more resistant to radiant energy than cotton or rayon, and these are less easily affected than nylon. Orlon appears to be appreciably more resistant than nylon. Fabrics of light weight (for a given area) need less thermal energy to cause specific damage than do those of heavy weight. The energy required, for the same exposure time, is roughly proportional to the fabric weight per unit area. Fabric with a moderate moisture content behaves like dry fabric, but if the amount of moisture is fairly high, more thermal energy will be needed to produce damage.
7.35 Although extensive studies have been made of the effects of thermal radiation on a large number of individual fabrics, it is difficult to summarize the results because of the many variables that have a significant influence. Some attempt is nevertheless made in Table 7.35 to give an indication of the magnitude of the exposures required to ignite (or otherwise damage) various fabric materials by the absorption of thermal radiation. The values are expressed in terms of gram-calories of thermal energy incident upon a 1 square centimeter area of material, i.e., cal/cm2, generally referred to as the “radiant exposure.” Results are presented for low air bursts with arbitrary energy yields of 35 kilotons, 1.4 megatons, and 20 megatons. It will be noted that, for the reasons given in § 7.30, the radiant exposure required to produce a particular effect increases with the yield.
| Radiant Exposure* (cal/cm2) | ||||||
|---|---|---|---|---|---|---|
| Material | Weight (oz/yd2 | Color | Effect on Material | 35 kilotons | 1.4 megatons | 20 megatons |
| CLOTHING FABRICS | ||||||
| Cotton | 8 | White | Ignites | 32 | 48 | 85 |
| Khaki | Tears on flexing | 17 | 27 | 34 | ||
| Khaki | Ignites | 20 | 30 | 39 | ||
| Olive | Tears on flexing | 9 | 14 | 21 | ||
| Olive | Ignites | 14 | 19 | 21 | ||
| Dark blue | Tears on flexing | 11 | 14 | 17 | ||
| Dark blue | Ignites | 14 | 19 | 21 | ||
| Cotton corduroy | 8 | Brown | Ignites | 11 | 16 | 22 |
| Cotton denim, new | 10 | Blue | Ignites | 12 | 27 | 44 |
| Cotton shirting | 3 | Khaki | Ignites | 14 | 21 | 28 |
| Cotton-nylon mixture | 5 | Olive | Tears on flexing | 8 | 15 | 17 |
| Olive | Ignites | 12 | 28 | 53 | ||
| Wool | 8 | White | Tears on flexing | 14 | 25 | 38 |
| Khaki | Tears on flexing | 14 | 24 | 34 | ||
| Olive | Tears on flexing | 9 | 13 | 19 | ||
| Dark blue | Tears on flexing | 8 | 12 | 18 | ||
| 20 | Dark blue | Tears on flexing | 14 | 20 | 26 | |
| Rainwear (double neoprene-coated nylon twill) | 9 | Olive | Begins to melt | 5 | 9 | 13 |
| Olive | Tears on flexing | 8 | 14 | 22 | ||
| DRAPERY FABRICS | ||||||
| Rayon gabardine | 6 | Black | Ignites | 9 | 20 | 26 |
| Rayon-acetate drapery | 5 | Wine | Ignites | 9 | 22 | 28 |
| Rayon gabardine | 7 | Gold | Ignites | ** | 24† | 28† |
| Rayon twill lining | 3 | Black | Ignites | 7 | 17 | 25 |
| Rayon twill lining | 3 | Beige | Ignites | 13 | 20 | 28 |
| Acetate-shantung | 3 | Black | Ignites | 10† | 22† | 35† |
| Cotton heavy draperies | 13 | Dark colors | Ignites | 15 | 18 | 34 |
| TENT FABRICS | ||||||
| Canvas (cotton) | 12 | White | Ignites | 13 | 28 | 51 |
| Canvas | 12 | Olive drab | Ignites | 12 | 18 | 28 |
| OTHER FABRICS | ||||||
| Cotton chenille bedspread | Light blue | Ignites | ** | 11† | 15† | |
| Cotton venetian blind tape, dirty | White | Ignites | 10 | 18 | 22 | |
| Cotton venetian blind tape | White | Ignites | 13† | 27† | 31† | |
| Cotton muslin window shade | 8 | Green | Ignites | 7 | 13 | 19 |
7.36 Since the shape and duration of the thermal pulse depend on the actual burst altitude, as well as on the yield, the radiant exposures given in Table 7.35 for “low air bursts” are somewhat approximate. In general, however, the radiant exposures in the three columns would apply to nuclear explosions below 100,000 feet altitude for which the times to the second maximum in the fireball temperature are 0.2, 1.0, and 3.2 seconds, respectively (§ 7.85).
7.37 Wood is charred by exposure to thermal radiation, the depth of the char being closely proportional to the radiant exposure. For sufficiently large amounts of energy per unit area, wood in some massive forms may exhibit transient flaming but persistent ignition is improbable under the conditions of a nuclear explosion. However, the transitory flame may ignite adjacent combustible material which is not directly exposed to the radiation. In a more-or-less finely divided form, such as sawdust, shavings, or excelsior, or in a decayed, spongy (punk) state, wood can be ignited fairly readily by the thermal radiation from a nuclear explosion, as will be seen below.
7.38 Roughly speaking, something like 10 to 15 calories per square centimeter of thermal energy are required to produce visible charring of unpainted and unstained pine, douglas fir, red wood, and maple. Dark staining increases the tendency of the wood to char, but light-colored paints and hard varnishes provide protection.2
7.39 Glass is highly resistant to heat, but as it is very brittle it is sometimes replaced by transparent or translucent plastic materials or combined with layers of plastic, as in automobile windshields, to make it shatterproof. These plastics are organic compounds and so are subject to decomposition by heat. Nevertheless, many plastic materials, such as Bakelite, cellulose acetate, Lucite, Plexiglas, polyethylene, and Teflon, have been found to withstand thermal radiation remarkably well. At least 60 to 70 cal/cm2 of thermal energy are required to produce surface melting or darkening.
RADIANT EXPOSURES FOR IGNITION OF VARIOUS MATERIALS
7.40 Studies have been made in laboratories and at nuclear tests of the radiant exposures required for the ignition of various common household items and other materials of interest. The results for low air bursts with three arbitrary yields are presented in Table 7.40; the conditions and limitations noted in § 7.36 also hold here. The radiant exposures given would be applicable to explosions at altitudes below 100,000 feet.
| Radiant Exposure* (cal/cm2) | ||||||
|---|---|---|---|---|---|---|
| Material | Weight (oz/yd2 | Color | Effect on Material | 35 kilotons | 1.4 megatons | 20 megatons |
| HOUSEHOLD TINDER MATERIALS | ||||||
| Newspaper, shredded | 2 | Ignites | 4 | 6 | 11 | |
| Newspaper, dark picture area | 2 | Ignites | 5 | 7 | 12 | |
| Newspaper, printed text area | 2 | Ignites | 6 | 8 | 15 | |
| Crepe paper | 1 | Green | Ignites | 6 | 9 | 16 |
| Kraft paper | 3 | Tan | Ignites | 10 | 13 | 20 |
| Bristol board, 3 ply | 10 | Dark | Ignites | 16 | 20 | 40 |
| Kraft paper carton, used (fiat side) | 16 | Brown | Ignites | 16 | 20 | 40 |
| New bond typing paper | 2 | White | Ignites | 24† | 30† | 50† |
| Colton rags | Black | Ignites | 10 | 15 | 20 | |
| Rayon rags | Black | Ignites | 9 | 14 | 21 | |
| Colton string scrubbing mop (used) | Gray | Ignites | 10† | 15† | 21† | |
| Colton string scrubbing mop (weathered) | Cream | Ignites | 10† | 19† | 26† | |
| Paper book matches, blue head exposed | Ignites | 11† | 14† | 20† | ||
| Excelsior, ponderosa pine | 2 lb/ft3 | Light yellow | Ignites | ** | 23† | 23† |
| OUTDOOR TINDER MATERIALS*** | ||||||
| Dry rotted wood punk (fir) | Ignites | 4† | 6† | 8† | ||
| Deciduous leaves (beech) | Ignites | 4 | 6 | 8 | ||
| Fine grass (cheat) | Ignites | 5 | 8 | 10 | ||
| Coarse grass (sedge) | Ignites | 6 | 9 | 11 | ||
| Pine needles, brown (ponderosa) | Ignites | 10 | 16 | 21 | ||
| CONSTRUCTION MATERIALS | ||||||
| Roll roofing, mineral surface | Ignites | ** | >34 | >116 | ||
| Roll roofing, smooth surface | Ignites | ** | 30 | 77 | ||
| Plywood, douglas fir | Flaming during exposure | 9 | 16 | 20 | ||
| Rubber, pale latex | Ignites | 50 | 80 | 110 | ||
| Rubber, black | Ignites | 10 | 20 | 25 | ||
| OTHER MATERIALS | ||||||
| Aluminum aircraft skin (0.020 in. thick) coated with 0.002 in. of standard white aircraft paint | Blisters | 15 | 30 | 40 | ||
| Colton canvas sandbags, dry filled | Failure | 10 | 18 | 32 | ||
| Coral sand | Explodes (popcorning) | 15 | 27 | 47 | ||
| Siliceous sand | Explodes (popcorning) | 11 | 19 | 35 | ||
RADIANT EXPOSURE AND SLANT RANGE
7.41 In order to utilize the data in Tables 7.35 and 7.40 to determine how far from the burst point, for an explosion of given energy yield, ignition of a particular material would be observed, it is required to know how the thermal energy varies with distance. For a specific explosion yield, the variation of radiation exposure with distance from the point of burst depends upon a number of factors, including the height of burst and the condition (or clarity) of the atmosphere. As seen earlier, the proportion of the total yield that appears as thermal energy and the character and duration of the thermal pulse vary with the height of burst. Furthermore, the height of burst and the atmospheric visibility determine the fraction of the thermal energy that can penetrate the atmosphere.
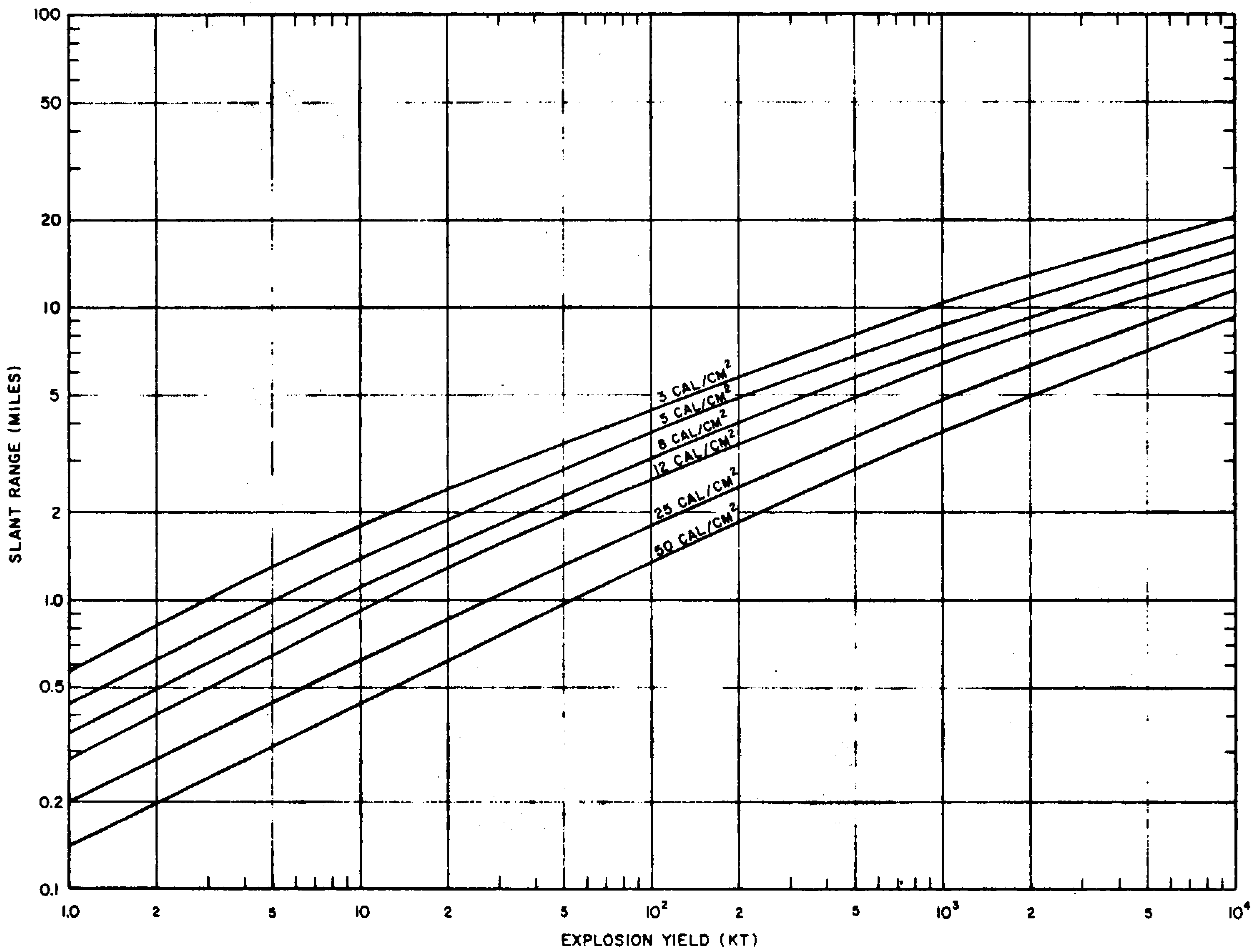
7.42 The variation of radiant exposure on the ground with slant range from the explosion for a particular set of conditions can be conveniently represented in the form of Fig. 7.42. These curves were calculated for burst heights of 200 feet, where is the explosion yield in kilotons (see § 7.99), but they provide reasonably good predictions of radiant exposures from air bursts at altitudes up to about 15,000 feet, for a visibility of 12 miles. This visibility represents the conditions for typical urban areas on a clear day. For air bursts at altitudes above 15,000 feet, Fig. 7.42 is not satisfactory and the procedures described in § 7.93 et seq. should be used. For bursts at low altitudes, e.g., less than 180 W0-4 feet, which are essentially surface bursts (cf. § 2.128), radiant exposures should be calculated by using the procedures in § 7.101 et seq.
7.43 The application of Fig. 7.42 may be illustrated by estimating the range over which ignition may occur in newspaper as a result of exposure to a 1000-kiloton (I-megaton) air burst under the conditions specified above. According to Table 7.40, the radiant exposure for the ignition of newspaper is about 8 cal/cm2 in a 1-megaton explosion. Fig. 7.42 is entered at the point on the yield scale corresponding to 1 megaton (103 kilotons); the perpendicular line is then followed until it intersects the curve marked 8 cal/cm2 of radiant exposure. The intersection is seen to correspond to a slant range of about 7 miles from the explosion. This is the range at which the thermal radiation from a 1-megaton air burst (below 15,000 feet altitude) could cause ignition in newspaper when the visibility is 12 miles. Under hazy conditions, such as often exist in large cities, the visibility would be less and the ignition range might be smaller. Similarly, a layer of dense cloud or smoke between the target and the burst point will decrease the distance over which a specified ignition may occur. However, if the explosion were to take place between-a cloud layer and the target or if the ground surface is highly reflective, as when covered with snow, the distance would be greater than indicated by Fig. 7.42.
THERMAL EFFECTS ON MATERIALS IN JAPAN3
7.44 Apart from the actual ignition of combustible materials resulting in fires being started, which will be referred to later, a number of other phenomena observed in Japan testified to the intense heat due to the absorption of thermal radiation. Fabrics (Fig. 7.44a), utility poles (Fig. 7.44b), trees, and wooden posts, up to a radius of 11,000 feet (2.1 miles) from ground zero to Nagasaki (estimated 3.4 cal/cm2 radiant exposure) and 9,000 feet (1.7 miles) at Hiroshima (estimated 3 cal/cm2), if not destroyed in the general conflagration, were charred and blackened, but only on the side facing the point of burst. Where there was protection by buildings, walls, hills, and other objects there was no evidence of thermal radiation effects. An interesting case of shadowing of this kind was recorded at Nagasaki. The tops and upper parts of a row of wooden posts were heavily charred, but the charred area was sharply limited by the shadow of a wall. The wall was, however, completely demolished by the blast wave which arrived after the thermal radiation. This radiation travels with the speed of light, whereas the blast wave advances much more slowly (§ 3.09).

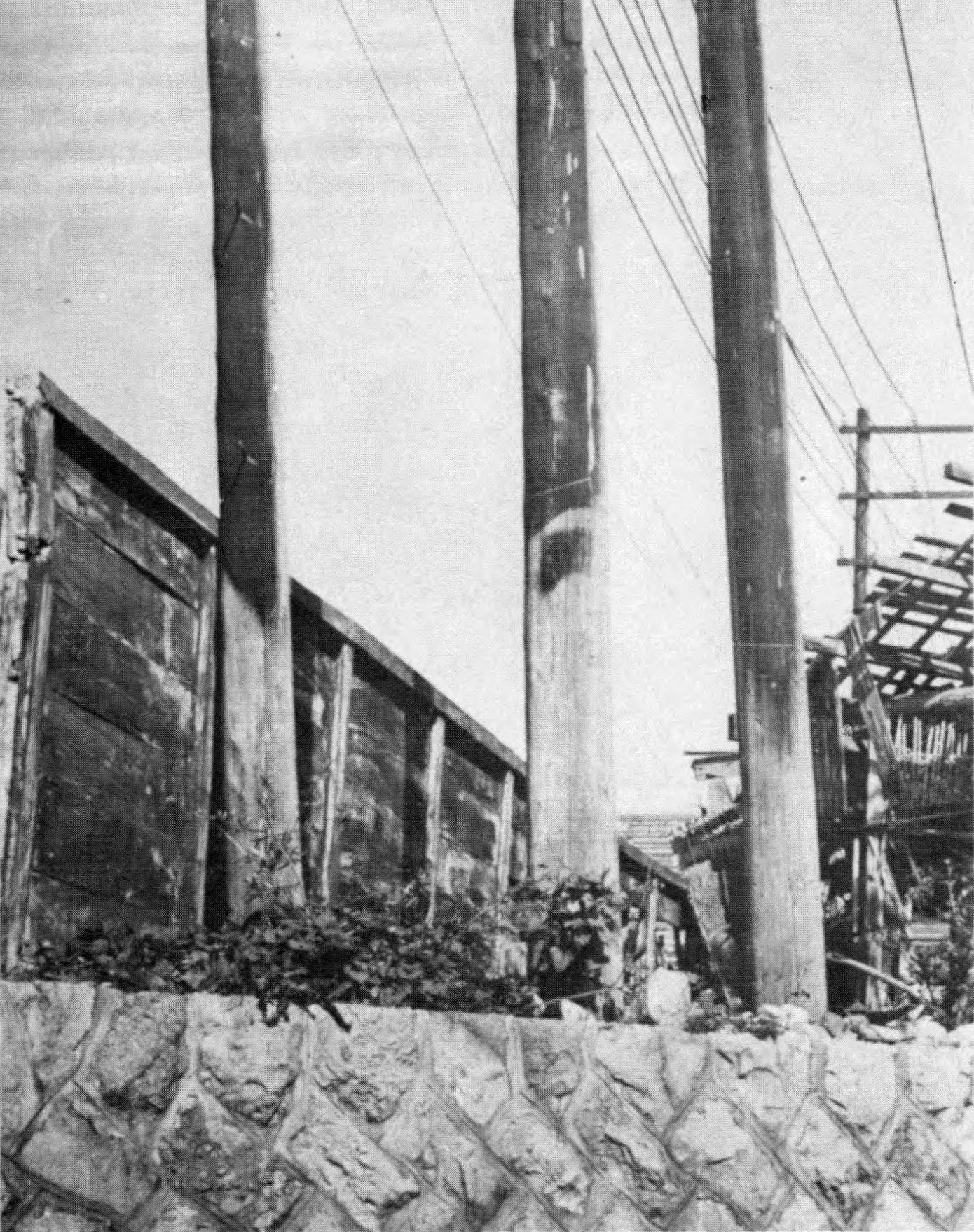
7.45 From observations of the shadows left by intervening objects where they shielded otherwise exposed surfaces (Figs. 7.45a and b), the direction of the center of the explosion was located with considerable accuracy. Furthermore, by examining the shadow effects at various places around the explosion, a good indication was obtained of the height of burst. Occasionally, a distinct penumbra was found, and from this it was possible to calculate the diameter of the fireball at the time the thermal radiation intensity was at a maximum.

7.46 One of the striking effects of the thermal radiation was the roughening of the surface of polished granite where there was direct exposure. This roughening was attributed to the unequal expansion of the constituent crystals of the stone, and it is estimated that a temperature of at least 600°C (1,100°F) was necessary to produce the observed effects. From the depth of the roughening and ultimate flaking of the granite surface, the depth to which this temperature was attained could be determined. These observations were used to calculate the maximum ground temperatures at the time of the explosion. As mentioned in § 7.26, they were extremely high, especially near ground zero.
7.47 Another thermal effect, which proved to be valuable in subsequent studies, was the bubbling or blistering of the dark green (almost black) tile with a porous surface widely used for roofing in Japan (Fig. 7.47). The phenomenon was reported as far as 3,200 feet (0.61 mile) from ground zero at Hiroshima, where the radiant exposure was estimated to have been 45 cal/cm2. The size of the bubbles and their extent increased with proximity to ground zero, and also with the directness with which the tile itself faced the explosion. In a laboratory test, using undamaged tile of the same kind, it was found that similar blistering could be obtained by heating to 1,800°C (3,270°F) for a period of 4 seconds, although the effect extended deeper into the tile than it did in Japan. From this result, it was concluded that in the nuclear explosion the tile attained a surface temperature of more than 1,800°C for a period of less than 4 seconds.

7.48 The difference in behavior of light and dark fabrics exposed to thermal radiation in Japan is also of considerable interest. Light-colored fabrics either reflect or transmit most of the thermal radiation and absorb very little. Consequently, they will not reach such a high temperature and will suffer less damage than dark fabrics which absorb a large proportion of the radiation. In one case, a shirt with alternate narrow light and dark gray stripes had the dark stripes burned out by a radiant exposure of about 7 cal/cm2, whereas the light-colored stripes were undamaged (Fig. 7.48). Similarly, a piece of paper which had received approximately 5 cal/cm2 had the characters, written in black ink, burned out, but the rest of the paper was not greatly affected.

INCENDIARY EFFECTS
ORIGIN OF FIRES
7.49 There are two general ways in which fires can originate in a nuclear explosion. First, as a direct result of the absorption of thermal radiation, thin kindling fuels can be ignited. And second, as an indirect effect of the destruction caused by the blast wave, fires can be started by upset stoves, water heaters, and furnaces, electrical short circuits, and broken gas lines. No matter how the fire originates, its subsequent spread will be determined by the amount and distribution of combustible materials in the vicinity.
7.50 In urban areas, kindling fuels which can be ignited by direct exposure to thermal radiation are located both indoors and out of doors. Interior ignition points could receive thermal radiation through a window or other opening. The thermal exposure at any interior point would be roughly proportional to the fraction of the fireball that would be visible at that point through the opening. If the thermal radiation should pass through a glass window, the amount entering a room would be about 80 percent of that falling on the exterior of the glass. The reduction is mainly due to reflection of the radiation, and so it is essentially independent of the thickness of the glass. A combination of a glass window and a screen will reduce the transmitted radiation energy to roughly 40 to 50 percent of the incident energy. In addition, the thermal radiation will be attenuated by window coverings, such as shades, curtains, and drapes. Of course, if the window coverings are made of combustible materials, they will constitute internal ignition points, as also will upholstered furniture, bedding, carpets, papers, and fabrics. Exterior ignition points are paper, trash, awnings, dry grass, leaves, and dry shrubs. Interior ignitions are more likely to grow into self-sustaining fires than are exterior ignitions. Large amounts of kindling are required to maintain an ignition for a sufficient time to ignite a sound wooden structure, and the necessary fuel arrangements are much more common indoors than outdoors.
7.51 In order for an ignition to develop within a room, one or two substantial combustible furnishings, such as an overstuffed chair or couch, a bed, or a wooden table, must be ignited and burn vigorously. Fires that become large enough to spread generally burn between 10 and 20 minutes before room “flashover.” Flashover occurs when flames from a localized fire suddenly spread to fill the room. After room flashover, the fire becomes intense enough to penetrate interior partitions and to spread to other rooms. The blaze from a single fire in an average residence may be expected to reach peak intensity in about an hour.
7.52 In a typical urban area the density of interior ignition points is usually much greater than that of exterior points. Furthermore, as stated above, the probability of ignitions spreading to more substantial fuels is greater for interior than for exterior ignition points. Nevertheless, fires started outdoors can also result in significant damage. Ignitions of dead weeds or tall dry grass or brush may develop into fires sufficiently intense to ignite houses. The fuel contained in a pile of trash is often sufficient to ignite a structure with loose, weathered siding. Structures with very badly weathered and decayed siding or shingles may ignite directly from the incident thermal radiation.
7.53 Since most of the thermal radiation reaches a target before the blast wave, the subsequent arrival of the latter may affect the development of fires initiated by the thermal radiation. In particular, there is a possibility that such fires may be extinguished by the blast wind. Studies of the effects in Japan and at various nuclear and high-explosive tests have given contradictory results and they leave the matter unresolved. Laboratory experiments that simulate blast loading of urban interiors show that the blast wave typically does extinguish flames but often leaves the material smoldering so that active flaming is revived at a later time. It is not certain, however, to what extent this behavior would apply to actual urban targets subjected to a nuclear explosion. Although some fires may be extinguished by the blast, many others will undoubtedly persist.
SPREAD OF FIRES
7.54 The spread of fires in a city, including the development of “mass fires,” depends upon various conditions, e.g., weather, terrain, closeness and combustibility of buildings, and the amount of combustible material in a given area. The interaction of blast and fire, as described above, and the extent of blast damage are also important factors in determining fire spread. Some conclusions concerning the development and growth of fires from a large number of ignition points were drawn from the experiences of World War II incendiary raids and the two nuclear bomb attacks on Japan, but these experiences were not completely documented. More useful data have been obtained from full-scale and model tests conducted in recent years.
7.55 The spread of fire between buildings can result from the ignition of combustible materials heated by fires in adjacent buildings, ignition of heated combustible materials by contact with flames, sparks, embers, or brands, and the ignition of unheated combustible materials by contact with flames or burning brands. Spread by heating, due either to convection, i.e., to the flow of hot gases, or to absorption of radiation, is a short-range effect, whereas spread by firebrands may be either short or long range. Hence, an important criterion of the probability of fire spread is the distance between buildings. The lower the building density, the less will be the probability that fire will spread from one structure to another. In an urban area, especially one fairly close to the explosion point, where substantial blast damage has occurred, the situation would be changed substantially. A deep, almost continuous layer of debris would cover the ground, thereby providing a medium for the ready spread of fires.
7.56 Combustible building surfaces exposed to a thermal radiation intensity of as low as 0.4 cal/cm2 per second for extended periods of time will ultimately burst into flame. The radiating portions of a burning building emit about 4 cal/cm2 per second. Consequently, radiation from a burning building may cause ignition of an adjacent building. Such ignition by radiation is probable for most structures if the dimensions of the burning structure are as large as, or larger than, the distance to the unburned structure. The convective plume of hot gases from a burning building would come into contact with another building which is farther away than the range for radiation fire spread only under conditions of extremely high wind. Therefore, fire spread by convective heat transfer is not expected to be a significant factor under normal terrain and weather conditions.
7.57 Fires can be spread between buildings by burning brands which are borne aloft by the hot gases and carried downwind for considerable distances. The fires can thus spread to distances greater than those at which radiative and convective heating can have a significant effect. Long-range fire spread by brands could greatly extend the area of destruction by urban fires resulting from nuclear explosions; there is no single method for predicting the spread but computer models are being developed for this purpose.
MASS FIRES
7.58 Under some conditions the many individual fires created by a nuclear explosion can coalesce into mass fires. The types of mass fires of particular interest, because of their great potential for destruction, are “firestorms” and conflagrations. In a firestorm many fires merge to form a single convective column of hot gases rising from the burning area and strong, fire-induced, radial (inwardly directed) winds are associated with the convective column. Thus the fire front is essentially stationary and the outward spread of fire is prevented by the in-rushing wind; however, virtually everything combustible within the fire storm area is eventually destroyed. Apart from a description of the observed phenomena, there is as yet no generally accepted definition of a fire storm. Furthermore, the conditions, e.g., weather, ignition-point density, fuel density, etc., under which a fire storm may be expected are not known. Nevertheless, based on World War II experience with mass fires resulting from air raids on Germany and Japan, the minimum requirements for a fire storm to develop are considered by some authorities to be the following: (1) at least 8 pounds of combustibles per square foot of fire area, (2) at least half of the structures in the area on fire simultaneously, (3) a wind of less than 8 miles per hour at the time, and (4) a minimum burning area of about half a square mile. High-rise buildings do not lend themselves to formation of fire storms because of the vertical dispersion of the combustible material and the baffle effects of the structures.
7.59 Conflagrations, as distinct from fire storms, have moving fire fronts which can be driven by the ambient wind. The fire can spread as long as there is sufficient fuel. Conflagrations can develop from a single ignition, whereas fire storms have been observed only where a large number of fires are burning simultaneously over a relatively large area.
7.60 Another aspect of fire spread is the development of mass fires in a forest following primary ignition of dried leaves, grass, and rotten wood by the thermal radiation. Some of the factors which will influence the growth of such fires are the average density and moisture content of the trees, the ratio of open to tree-covered areas, topography, season of the year, and meteorological conditions. Low atmospheric humidity, strong winds, and steep terrain favor the development of forest fires. In general, a deciduous forest, particularly when in leaf, may be expected to burn less rapidly and with less intensity than a forest of coniferous trees. Green leaves and the trunks of trees would act as shields against thermal radiation, so that the number of points at which ignition occurs in a forest may well be less than would appear at first sight.
INCENDIARY EFFECTS IN JAPAN
THE NUCLEAR BOMB AS AN INCENDIARY WEAPON
7.61 The incendiary effects of a nuclear explosion do not present any especially characteristic features. In principle, the same overall result, as regards destruction by fire and blast, might be achieved by the use of conventional incendiary and high-explosive bombs. It has been estimated, for ex ample, that the fire damage to buildings and other structures suffered at Hiroshima could have been produced by about 1,000 tons of incendiary bombs distributed over the city. It can be seen, however, that since this damage was caused by a single nuclear bomb of only about 12.5 kilotons energy yield, nu clear weapons are capable of causing tremendous destruction by fire, as well as by blast.
7.62 Evidence was obtained from the nuclear explosions over Japan that the damage by fire is much more dependent upon local terrain and meteorological conditions than are blast effects. At both Hiroshima and Nagasaki the distances from ground zero at which particular types of blast damage were experienced were much the same. But the ranges of incendiary effects were quite different. In Hiroshima, for example, the total area severely damaged by fire, about 4.4 square miles, was roughly four times as great as in Nagasaki. One contributory cause was the irregular layout of Nagasaki as compared with Hiroshima; also greater destruction could probably have been achieved by a change in the burst point. Nevertheless, an important factor was the difference in terrain, with its associated building density. Hiroshima was relatively flat and highly built up, whereas Nagasaki had hilly portions near ground zero that were bare of structures.
ORIGIN AND SPREAD OF FIRES IN JAPAN
7.63 Definite evidence was obtained from Japanese observers that the thermal radiation caused thin, dark cotton cloth, such as the blackout curtains that were in common use during the war, thin paper, and dry, rotted wood to catch fire at distances up to 3,500 feet (0.66 mile) from ground zero. It was reported that a cedar bark roof farther out was seen to burst into flame, apparently spontaneously, but this was not definitely confirmed. Abnormal enhanced amounts of radiation, due to reflection, scattering, and focusing effects, might have caused fires to originate at isolated points (Fig. 7.63).

7.64 From the evidence of charred wood found at both Hiroshima and Nagasaki, it was originally concluded that such wood had actually been ignited by thermal radiation and that the flames were subsequently extinguished by the blast. But it now seems more probable that, apart from some exceptional instances, there was no actual ignition of the wood. The absorption of the thermal radiation caused charring in sound wood but the temperatures were generally not high enough for ignition to occur (§ 7.28). Rotted and checked (cracked) wood and excelsior, however, have been observed to burn completely, and the flame was not greatly affected by the blast wave.
7.65 It is not known to what extent thermal radiation contributed to the initiation of fires in the nuclear bombings in Japan. It is possible, that, up to a mile or so from ground zero, some fires may have originated from secondary causes, such as upsetting of stoves, electrical short-circuits, broken gas lines, and so on, which were a direct effect of the blast wave. A number of fires in industrial plants were initiated by furnaces and boilers being overturned, and by the collapse of buildings on them.
7.66 Once the fires had started, there were several factors, directly related to the destruction caused by the nuclear explosion, that influenced their spreading. By breaking windows and blowing in or damaging fire shutters (Fig. 7.66), by stripping wall and roof sheathing, and collapsing walls and roofs, the blast made many buildings more vulnerable to fire. Noncombustible (fire-resistive) structures were often left in a condition favorable to the internal spread of fires by damage at stairways, elevators, and in firewall openings as well as by the rupture and collapse of floors and partitions (see Fig. 5.23).

7.67 On the other hand, when combustible frame buildings were blown down, they did not burn as rapidly as they would have done had they remained standing. Moreover, the non combustible debris produced by the blast frequently covered and prevented the burning of combustible material. There is some doubt, therefore, whether on the whole the effect of the blast was to facilitate or to hinder the development of fires at Hiroshima and Nagasaki.
7.68 Although there were fire breaks, both natural, e.g., rivers and open spaces, and artificial, e.g., roads and cleared areas, in the Japanese cities, they were not very effective in preventing the fires from spreading. The reason was that fires often started simultaneously on both sides of the firebreaks, so that they could not serve their intended purpose. In addition, combustible materials were frequently strewn by the blast across the firebreaks and open spaces, such as yards and street areas, so that they could not prevent the spread of fires. Nevertheless, there were a few instances where firebreaks assisted in preventing the burnout of some fire-resistive buildings.
7.69 One of the important aspects of the nuclear attacks on Japan was that, in the large area that suffered simultaneous blast damage, the fire departments were completely overwhelmed. It is true that the fire-fighting services and equipment were poor by American standards, but it is doubtful if much could have been achieved, under the circumstances, by more efficient fire departments. At Hiroshima, for example, 70 percent of the firefighting equipment was crushed in the collapse of fire houses, and 80 percent of the personnel were unable to respond. Even if men and machines had survived the blast, many fires would have been inaccessible because of the streets being blocked with debris. For this reason, and also because of the fear of being trapped, a fire company from an area which had escaped destruction was unable to approach closer than 6,600 feet (1.25 miles) from ground zero at Nagasaki.
7.70 Another contributory factor to the destruction by fire was the failure of the water supply in both Hiroshima and Nagasaki. The pumping stations were not largely affected, but serious damage was sustained by distribution pipes and mains, with a resulting leakage and drop in available water pressure. Most of the lines above ground were broken by collapsing buildings and by heat from the fires which melted the pipes. Some buried water mains were fractured and others were broken due to the collapse or distortion of bridges upon which they were supported (§ 5.106).
7.71 About 20 minutes after the detonation of the nuclear bomb at Hiroshima, a mass fire developed showing many characteristics usually associated with fire storms. A wind blew toward the burning area of the city from all directions, reaching a maximum velocity of 30 to 40 miles per hour about 2 to 3 hours after the explosion, decreasing to light or moderate and variable in direction about 6 hours after. The wind was accompanied by intermittent rain, light over the center of the city and heavier about 3,500 to 5,000 feet (0.67 to O.95 mile) to the north and west. Rain in these circumstances was apparently due to the condensation of moisture on particles from the fire when they reached a cooler area. The strong inward draft at ground level was a decisive factor in limiting the spread of fire beyond the initial ignited area. It accounts for the fact that the radius of the burned-out area was so uniform in Hiroshima and was not much greater than the range in which fires started soon after the explosion. However, virtually everything combustible within this region was destroyed.
7.72 No definite fire storm occurred at Nagasaki, although the velocity of the southwest wind blowing between the hills increased to 35 miles an hour when the conflagration had become well established, perhaps about 2 hours after the explosion. This wind tended to carry the fire up the valley in a direction where there was nothing to burn. Some 7 hours later, the wind had shifted to the east and its velocity had dropped to 10 to 15 miles per hour. These winds undoubtedly restricted the spread of fire in the respective directions from which they were blowing. The small number of dwellings exposed in the long narrow valley running through Nagasaki probably did not furnish sufficient fuel for the development of a fire storm as compared to the many buildings on the flat terrain at Hiroshima.
TECHNICAL ASPECTS OF THERMAL RADIATION4
DISTRIBUTION AND ABSORPTION OF ENERGY FROM THE FIREBALL
7.73 Spectroscopic studies made in the course of weapons tests have shown that the fireball does not behave exactly like a black body, i.e., as a perfect radiator. Generally, the proportion of radiations of longer wavelength (greater than 5,500 A) corresponds to higher black body temperatures than does the shorter wave emission. The assumption of black body behavior for the fireball, however, serves as a reasonable approximation in interpreting the thermal radiation emission characteristics. For a black body, the distribution of radiant energy over the spectrum can be related to the surface temperature by Planck’s radiation equation. If denotes the energy density, i.e., energy per unit volume, in the wavelength interval to , then,
where is the velocity of light, is Planck’s quantum of action, is Boltzmann’s constant, i.e., the gas constant per molecule, and is the absolute temperature. It will be noted that is the energy of the photon of wavelength (§ 1.74).
7.74 From the Plank equation it is possible to calculate the rate of energy emission (or radiant power) of a black body for a given wavelength, i.e., , as a function of wavelength for any specified temperature, since is related to by
where is in units of energy (ergs) per unit area (cm2) per unit time (sec) per unit wavelength (A). The results of such calculations for temperatures ranging from 100 million (108) degrees to 2,000°K are shown in Fig. 7.74. It is seen that the total radiant power, which is given by the area under each curve, decreases greatly as the temperature is decreased.
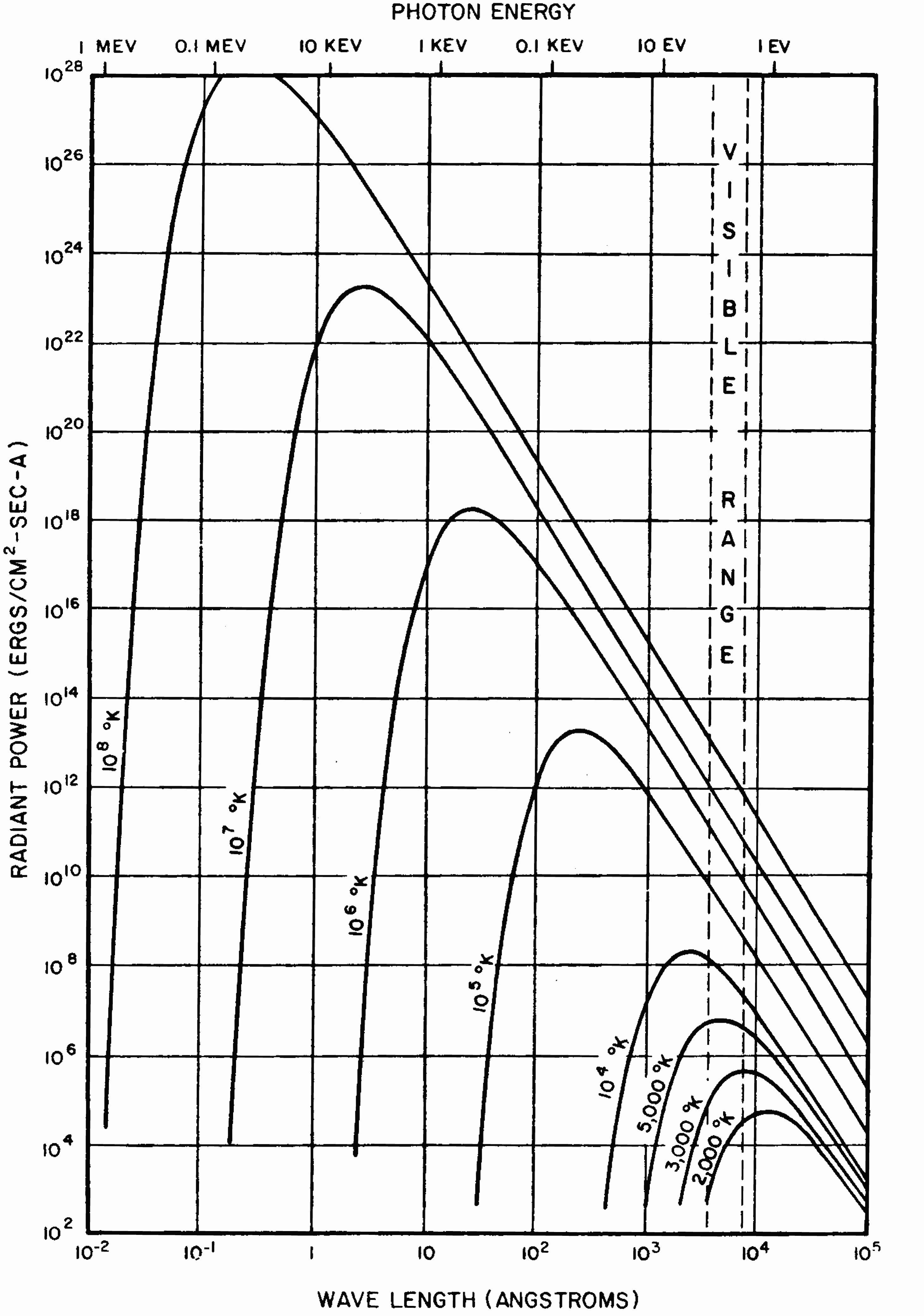
7.75 An important aspect of Fig. 7.74 is the change in location of the curves with temperature; in other words, the spectrum of the radiant energy varies with the temperature. At high temperatures, radiations of short wavelength predominate, but at low temperatures those of long wavelength make the major contribution. For example, in the exploding weapon, before the formation of the fireball, the temperature is several tens of million degrees Kelvin. Most of the (primary) thermal radiation is then in the wavelength range from about 0.1 to 100 A, i.e., 120 to 0.12 kilo-electron volts (keV) energy. corresponding roughly to the soft X-ray region (Fig. l.74). This is the basis of the statement made earlier that the primary thermal radiation from a nuclear explosion consists largely of X rays. These radiations are absorbed by the surrounding air to form the fireball from which the effective thermal radiation of present interest is emitted in the ultraviolet, visible, and infrared regions of the spectrum. The dimensions of the fireball in which the thermal X rays are absorbed depends on the ambient air density, as will be seen shortly.
7.76 It will be recalled that the thermal radiation received at the earth’s surface differs to some extent from that leaving the fireball. The reason is that the radiations. of shorter wavelength, i.e., in the ultraviolet, are more readily absorbed than the others by the atmosphere between the burst point and the earth’s surface. The thermal radiation received at a distance from a nuclear explosion is fairly characteristic of a black body at a temperature of about 6,000 to 7,000°K, although somewhat depleted in the ultraviolet and other shorter wavelengths. Even if the detonation occurs at very high altitudes, the thermal radiation from the low-density fireball must pass through the denser atmosphere before reaching the ground. The effective thermal radiation received on the earth’s surface in this case is, therefore, also composed of the longer wavelengths.
7.77 An expression for the wave length () corresponding to the maximum in the radiant power as a function of the black body temperature can be obtained by differentiating equation (7.74.1) with respect to wavelength and equating the result to zero. It is then found that
where is a constant, equal to angstroms-degrees K. This expression is known as Wien’s displacement law.
7.78 The temperature at which the maximum in the radiant power distribution from a black body should just fall into the visible spectrum, i.e., wave length 3,850 A, is found from equation (7.77. l) to be about 7,500°K. This happens to be very close to the maximum surface temperature of the fireball after the minimum, i.e., during the second radiation pulse (Fig. 2.39). Since the apparent surface temperature generally does not exceed 8,000°K and the average is considerably less, it is evident that the thermal energy emitted in the second pulse should consist mainly of visible and infrared rays, with a smaller proportion in the ultraviolet region of the spectrum. This has been found to be the case in actual tests, even though the fireball deviates appreciably from black body behavior at this stage.
7.79 The mean free path (§ 2.113) in cold air, at sea-level density, of X-ray photons with energies from about 0.5 to 15 keV is given by the approximate relationship
where is the photon energy in keV. In order to make some order-of-magnitude calculations of the distances in which thermal X rays from a nuclear explosion are absorbed in air, a convenient round number temperature of 107 degrees Kelvin will be used for simplicity. From equation (7.77. l), the wavelength at which the rate of emission of radiation from a black body at this temperature is a maximum is found to be 2.9 A. According to equation (1.74.2) this corresponds to a photon energy of 4.3 keV, and from equation (7.79.1) the mean free path of these photons in normal air is about 15 cm. In traversing a distance of one mean free path the energy of the radiations decreases by a factor of e, i.e., approximately 2.7; hence 90 per cent of the energy will be deposited within a radius of 2.3 mean free paths. It is seen, therefore, that 4.3-keV radiation will be largely absorbed in a distance of about 35 cm, i.e., a little over 1 foot, in a sea-level atmosphere.
7.80 The primary thermal radiations from a nuclear explosion cover a wide range of wavelengths, as is evident from Fig. 7.74. But to obtain a rough indication of the initial size of the fire ball, the wavelength (or energy) at which the radiant power from a black body is a maximum may be taken as typical. It follows, therefore, from the results given above that the thermal X rays from a nuclear explosion will be almost completely absorbed by about a foot of air at normal density. The oxygen and nitrogen in the air in the vicinity of the explosion are considerably ionized, and the ions do not absorb as effectively as do neutral molecules. Nevertheless, in a nuclear explosion in the atmosphere where the air density does not differ greatly from the sea-level value, most of the X rays, which constitute the primary thermal radiation, will be absorbed within a few feet of the explosion. It is in this manner that the initial fireball is formed in an air burst.
7.81 With increasing altitude, the air density decreases roughly by a factor of ten for every 10 miles (see § 10.124); hence at 155,000 feet (approximately 30 miles), for example, the density is about 10-3 of the sea-level value. The mean free path of the photon varies inversely as the density, so that for nuclear explosions at an altitude of about 30 miles, the region of the air heated by X rays, which is equivalent to the fireball, extends over a radius of some thousands of feet. In spite of the lower density, the mass of heated air in this large volume is much greater than in the fireball associated with a nuclear explosion at lower altitudes, and so the temperature attained by the air is lower.
THERMAL POWER AND ENERGY FROM THE FIREBALL
7.82 According to the Stefan Boltzmann law, the total amount of energy (of all wavelengths), , radiated per square centimeter per second by a black body in all directions in one hemisphere is related to the absolute temperature, , by the equation
where is the Stefan-Boltzmann constant. The value of can also be obtained by integration of equation (7.74.1) over all wavelengths from zero to infinity. It is then found that
With a known, the total radiant energy intensity from the fireball behaving as a black body can be readily calculated for any required temperature.
7.83 In accordance with the definition of , given above, it follows that the total rate of emission of radiant energy from the fireball can be obtained upon multiplying the expression in equation (7.82.1) by the area. If is the radius of the fireball, its area is , so that the total rate of thermal energy emission (or total radiant power) is . Representing this quantity by the symbol , it follows that
where is in degrees Kelvin and is in centimeters. Alternatively, if the radius, , is expressed in feet, then
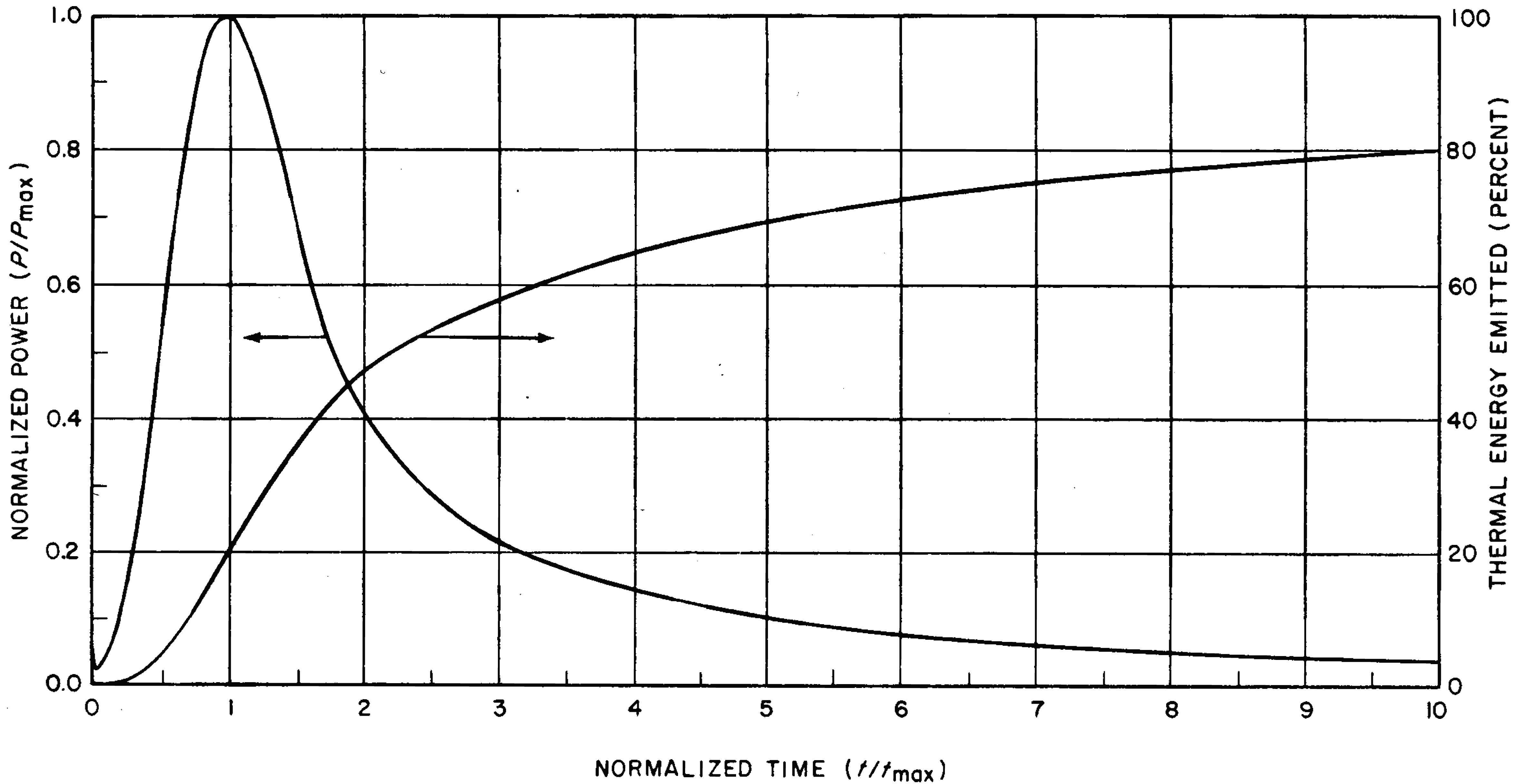
7.84 Complex interactions of hydrodynamic and radiation factors govern the variation of the apparent size and temperature of the fireball with time. Nevertheless, the fireball thermal power can be calculated as a function of time based upon theoretical considerations modified by experimental measurements. The results are conveniently expressed as the scaled power, i.e., , versus the scaled time, i.e., ; is the thermal power at any time after the explosion, and the is the maximum value of the thermal power at the time, , of the second temperature maximum (§ 2.125). The resulting (left scale) curve, shown in Fig. 7.84, is then of general applicability irrespective of the yield of the explosion. Changes in yield and altitude can affect the shape of the power pulse; however, the values in Fig. 7.84 are reasonably accurate for most air bursts below 100,000 feet. The zero of the scaled time axis is the time of the first maximum, but for all practical purposes this may be taken as the explosion time.
THERMAL ENERGY FROM AN AIR BURST
7.85 In order to make the power time curve specific for any particular explosion energy yield, it is necessary to know the appropriate values of and Theoretically, these quantities should depend on the air density, but experimental evidence indicates that the dependence is small for air bursts at altitudes below 15,000 feet; in this altitude range, and are related approximately to the yield, kilotons, in the following manner:
For heights of burst above 15,000 feet the data are sparse. Theoretical calculations indicate that the corresponding relationships are as follows:
In these expressions is the ambient air density at the burst altitude and is the normal ambient air density at sea level (taken to be 1.225 x 10-3 gram/cm3). Values of are given in Table 7.85 for several altitudes. Use of the preceding equations results in a discontinuity at 15,000 feet. For heights of burst at or near that altitude, values should be calculated by both sets of equations, and the appropriate result should be used depending on whether offensive or defensive conservatism is desired. For a contact surface burst (§ 2.127 footnote) the fireball develops in a manner approaching that for an air burst of twice the yield, because the blast wave energy is reflected back from the surface into the fireball (§ 3.34). Hence, may be expected to be larger than for an air burst of the same actual yield.
| Altitude (feet) | Density Ratio, p(h)/po | Altitude (feet) | Density Ratio, p(h)/po |
|---|---|---|---|
| 15,000 | 0.63 | 60,000 | 0.095 |
| 20,000 | 0.53 | 65,000 | 0.075 |
| 25,000 | 0.45 | 70,000 | 0.059 |
| 30,000 | 0.37 | 75,000 | 0.046 |
| 35,000 | 0.31 | 80,000 | 0.036 |
| 40,000 | 0.24 | 85,000 | 0.028 |
| 45,000 | 0.19 | 90,000 | 0.022 |
| 50,000 | 0.15 | 95,000 | 0.017 |
| 55,000 | 0.12 | 100,000 | 0.014 |
7.86 The thermal power curve in Fig. 7.84 (left scale) presents some features of special interest. As is to be expected, the thermal power (or rate of emission of radiant energy) of the fire ball rises to a maximum, just as does the temperature in the second radiation pulse. However, since the thermal power is roughly proportional to , it increases and decreases much more rapidly than does the temperature. This accounts for the sharp rise to the maximum in the curve, followed by a somewhat less sharp drop which tapers off as the fireball approaches its final stages. The amount of thermal energy, , emitted by the fireball in an air burst up to any specified time can be obtained from the area under the curve of versus up to that time. The results, expressed as (percent) versus , are shown by the second curve (right scale) in Fig. 7.84, where is the total thermal energy emitted by the fireball. It is seen that at a time equal to 10 about 80 percent of the thermal energy will have been emitted; hence this time may be taken as a rough measure of the effective duration of the thermal pulse for an air burst. Since increases with the explosion energy yield, so also does the pulse length.
7.87 The fact that the thermal pulse length increases with the weapon yield has a bearing on the possibility of people taking evasive action against thermal radiation. Evasive action is expected to have greater relative effectiveness for explosions of higher than lower yield because of the longer thermal pulse duration. The situation is indicated in another way in Fig. 7.87, which shows the thermal energy emission as a function of actual time, rather than of for four different explosion energy yields. The data were derived from the corresponding curve in Fig. 7.84 by using the appropriate calculated value of for each yield. At the lower energy yields the thermal radiation is emitted in such a short time that no evasive action is possible. At the higher yields, however, exposure to much of the thermal radiation could be avoided if evasive action were taken within a fraction of a second of the explosion time. It must be remembered, of course, that even during this short period a very considerable amount of thermal energy will have been emitted from an explosion of high yield.
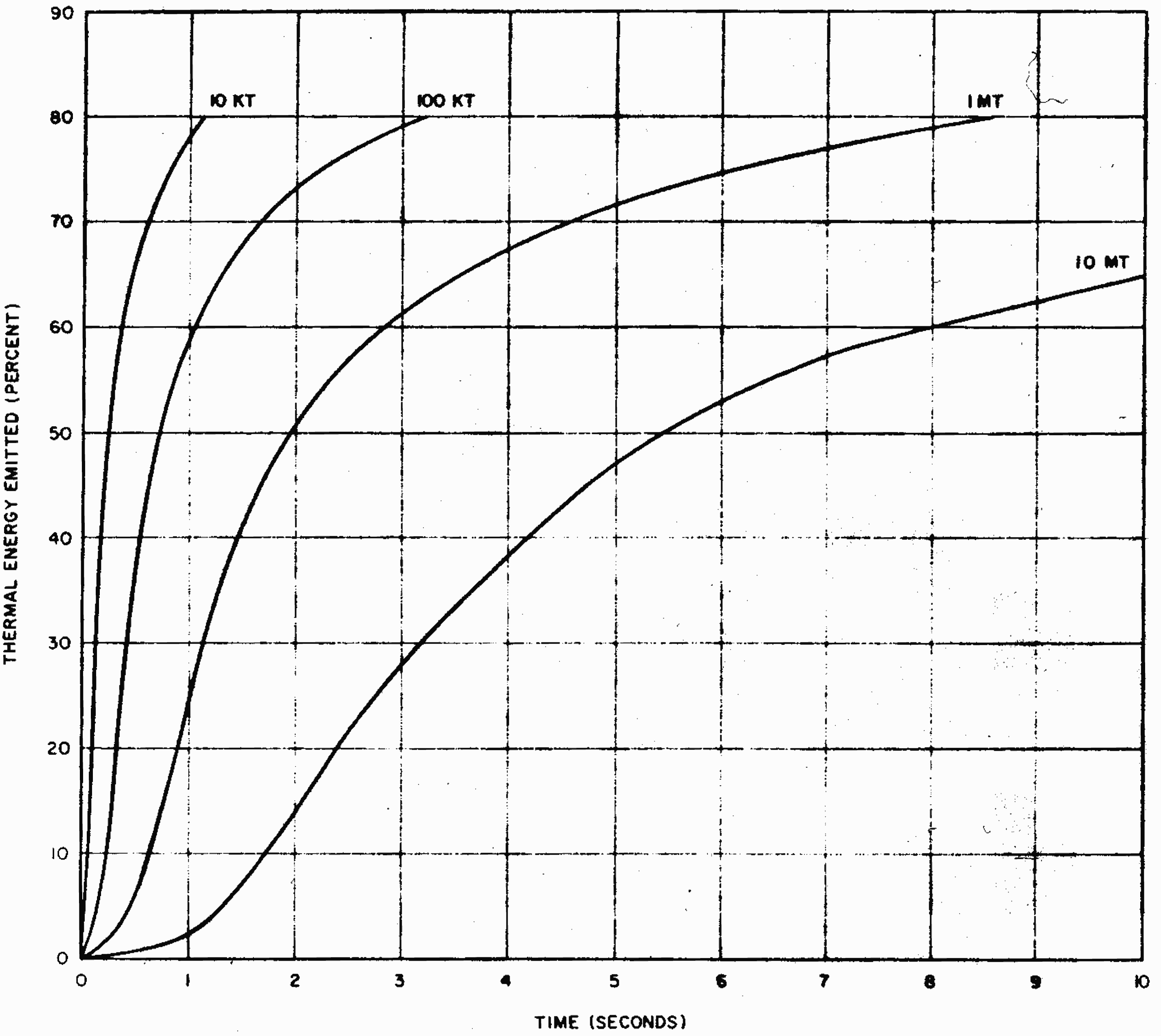
7.88 The fraction of the explosion energy yield in the form of thermal radiation, i.e., , is called the “thermal partition” and is represented by the symbol . Estimated values of are given in Table 7.88 for air bursts with yields in the range from 1 kiloton to 10 megatons at altitudes up to 100,000 feet (19 miles). The data for heights of burst up to 15,000 feet were obtained primarily from experimental results. For higher bursts altitudes, the values were obtained by calculations, various aspects of which were checked with experimental results. They are considered to be fairly reliable for yields between 1 kiloton and 1 megaton at altitudes up to 50,000 feet (9.5 miles). Outside this range of yields and altitudes, the data in Table 7.88 may be used with less confidence. Values of for burst altitudes above 100,000 feet are given in § 7.90 (see also§ 7.104).
| Thermal Partition, f | |||||
|---|---|---|---|---|---|
| Total Yield (kilotons) | |||||
| Height of Burst (kilofeet) | 1 | 10 | 100 | 1,000 | 10,000 |
| Up to 15 | 0.35 | 0.35 | 0.35 | 0.35 | 0.35 |
| 20 | 0.35 | 0.36 | 0.39 | 0.41 | 0.43 |
| 30 | 0.35 | 0.36 | 0.39 | 0.41 | 0.43 |
| 40 | 0.35 | 0.36 | 0.38 | 0.40 | 0.42 |
| 50 | 0.35 | 0.36 | 0.38 | 0.40 | 0.42 |
| 60 | 0.35 | 0.37 | 0.38 | 0.40 | 0.42 |
| 70 | 0.36 | 0.37 | 0.39 | 040 | 0.42 |
| 80 | 0.37 | 0.38 | 0.39 | 0.41 | 0.43 |
| 90 | 0.38 | 0.39 | 0.40 | 0.41 | 0.43 |
| 100 | 0.40 | 0.40 | 0.41 | 0.42 | 0.45 |
THERMAL RADIATION IN HIGH-ALTITUDE EXPLOSIONS
7.89 The results described above are applicable to detonations at altitudes below about 100,000 feet (19 miles) where the density of the air is still appreciable. At higher altitudes, the fire ball phenomena change, as described in Chapter II, and so also do the thermal pulse characteristics, such as shape and length, and the thermal partition. With increasing altitude, there is a tendency for the relative duration of the first pulse, i.e., up to the first temperature minimum (§§ 2.39, 2.125), to increase and for the minimum to be less marked. Up to an altitude of about 100,000 feet, these changes are small and so also are those in the second thermal pulse. The normalized plot (Fig. 7.84) is thus a satisfactory representation in this altitude range. However, between 100,000 and 130,000 feet (25 miles), the pulse shape changes drastically. The first minimum observed at lower altitudes disappears and essentially all the thermal radiation is emitted as a single pulse (§ 2.132). The thermal emission rises to a maximum in an extremely short time and then declines steadily, at first rapidly and later more slowly. For an ex plosion in the megaton range at an altitude of 250,000 feet (about 47 miles), the duration of the thermal pulse is less than a second compared with a few seconds for a similar burst below 100,000 feet (cf. Fig. 7.87). Scaling of the pulse length with respect to the explosion yield at high altitudes is very complex and depends on a variety of factors. However, the duration of the thermal pulse is probably not strongly dependent on the total yield. At altitudes above roughly 270,000 feet (51 miles), the pulse length increases because of the larger mass and lower temperature of the radiating region (§ 7.91).
7.90 At high altitudes shock waves form much less readily in the thinner air and consequently the fireball is able to radiate thermal energy that would, at lower altitudes, have been transformed to hydrodynamic energy of the blast wave. Furthermore, the thinner air allows the primary thermal radiation (X rays) from the explosion to travel much farther than at lower levels. Some of this radiation travels so far from the source that it makes no contribution to the energy in the fireball. Between about 100,000 and 160,000 feet (30 miles), the first factor is dominant and the proportion of energy in the blast wave decreases; consequently, the thermal energy increases. In this altitude range the thermal partition, , is about 0.6, compared with 0.40 to 0.45 at 100,000 feet (Table 7.88). Above 160,000 feet, however, the second factor, i.e., escape of thermal X rays, becomes increasingly important; the thermal partition de creases to about 0.25 at 200,000 feet (38 miles) and remains at this value up to roughly 260,000 feet (49 miles). At still higher altitudes there is a change in the fireball behavior (§ 2.135) and the thermal partition decreases very rapidly with increasing altitude of the explosion.
7.91 At heights of burst above about 270,000 feet, only the primary X rays traveling downward are absorbed and the energy deposition leads to the formation of the incandescent X-ray pancake described in Chapter II. This heated region then reradiates its energy at longer wavelengths over a period of several seconds. The altitude and dimensions of the pancake depend to some extent on the explosion yield but, as stated in § 2.134, reasonable average values are 30,000 feet for the thickness, 270,000 feet for the mean altitude, and the height of burst minus 270,000 feet for the radius at this altitude. The altitude and thickness of the reradiating region are essentially independent of the height of the burst above 270,000 feet, but the mean radius increases with the burst height. The shape of the region thus approaches a thick disk (or frustum) centered at about 270,000 feet altitude.
7.92 Not more than one-fourth of the X-ray energy from the explosion is absorbed in the low-density air of the reradiating region, and only a small fraction, which decreases with increasing height of burst, is reradiated as secondary radiation. Consequently, only a few percent of the weapon energy is emitted as thermal radiation capable of causing damage at the earth’s surface. In fact, for bursts at altitudes exceeding some 330,000 feet (63 miles), the thermal radiation from a nuclear explosion even in the megaton range is essentially ineffective so far as skin burns, ignition, etc., are concerned. However, the early-time debris, which separates from the X-ray pancake (§ 2.135), is at a fairly high temperature and it emits a very short pulse of thermal energy that can cause eye injury to individuals looking directly at the explosion (§ 12.79 et seq.).
RADIANT EXPOSURE-DISTANCE RELATIONSHIPS
AIR BURSTS
7.93 The following procedure is used to calculate the dependence of the radiant exposure of a target (§ 7.35) upon its distance from an air burst of specified yield. As seen earlier in this chapter, such information, which is given in Fig. 7.42, combined with the data in Tables 7.35 and 7.40, permits estimates to be made of the probable ranges for various thermal radiation effects.
7.94 If there is no atmospheric attenuation, then at a distance from the explosion the thermal radiation energy, , may be regarded as being spread uniformly over the surface of a sphere of area . If the radiating fireball is treated as a point source, the energy received per unit area of the sphere would be . If attenuation were due only to absorption in a uniform atmosphere, e.g., for an air burst, this quantity would be multiplied by the factor , where κ is an absorption coefficient averaged over the whole spectrum of wavelengths. Hence in these circumstances, using the symbol to represent the radiant exposure, i.e., the energy received per unit area normal to the direction of propagation, at a distance D from the explosion, it follows that
7.95 When scattering of the radiation occurs, in addition to absorption, the coefficient κ changes with distance and other variables. The simple exponential attenuation factor in equation (7.94.1) is then no longer adequate. A more useful (empirical) formulation is
where the transmittance, , i.e., the fraction of the radiation (direct and scattered) which is transmitted, is a complex function of the visibility (scattering), absorption, and distance.5
7.96 Since , equation (7.95.1) for the radiant exposure from an air burst of yield can be expressed as
By utilizing the fact that 1 kiloton of TNT is equivalent to 1012 calories, equation (7.96.1) for an air burst becomes
where is in centimeters and is in kilotons. If the distance, , from the explosion to the target, i.e., the slant range, is expressed in kilofeet or miles, equation (7.96.2) reduces approximately to
7.97 In nuclear weapons tests, it is possible to measure and , and since the distance from the explosion is known the magnitude of the product can be determined from the equations in § 7.96. Hence, to obtain and individually, one of these two quantities must be determined independently of the other. The method used is to obtain for different conditions from calculations checked by observations, as stated in § 7.88. The values of are then derived from measurements of made at a large number of weapons tests.
7.98 The transmittance for any given atmospheric condition depends on the solid angle over which scattered radiation can reach a particular exposed object. For the present purpose it will be assumed that the target is such, e.g., an appreciable flat area, that scattered radiation is received from all directions above, in addition to the direct thermal radiation from the source. Transmittance data for these conditions are presented in Fig. 7.98 in terms of burst altitude and distance of a surface target from ground zero for a cloudless atmosphere with a visibility of 12 miles. Since actual visibilities in cities are often less, the values in Fig. 7.98 are conservative.
7.99 The transmittance values in Fig. 7.98 were used, in conjunction with equation (7.96.4) and the thermal partitions from Table 7.88, to obtain the data from which the curves in Fig. 7.42 were constructed. If is the height of burst and is the distance from ground zero of a given point on the surface, the corresponding slant range for use in equation (7.96.4) is . A height of burst of 200 feet, with in kilotons, was used for the calculations, but the results in Fig. 7.42 are reasonably accurate for air bursts at any altitude up to some 15,000 feet.
7.100 Under unusual conditions and especially for cities at high-altitude locations, the visibility might be greater than at sea level and the transmittance would be larger than the values given in Fig. 7.98. The curves show that most attenuation of radiation occurs within a few thousand feet of the surface; thus, the much clearer air at higher altitudes has less effect. For bursts above about 150,000 feet (28 miles), the transmittance changes slowly with the altitude. Experimental data indicate that multiplying the transmittance by 1.5 corrects approximately for the effect of reflection from a cloud layer over the burst. The same correction may be made for a snow-covered ground surface. If the burst and target are both between a cloud layer and a snow covered surface, the correction is 1.5 x 1.5 = 2.25.
SURFACE BURSTS
7.101 For a surface burst, the radiant exposures along the earth’s surface will be less than for equal distances from an air burst of the same total yield. This difference arises partly, as indicated in § 7.20, from the decreased transmittance of the intervening low air layer due to dust and water vapor produced by the explosion. Furthermore, the normal atmosphere close to the earth’s surface transmits less than at higher altitudes. In order to utilize the equations in § 7.96 to determine radiant exposure for surface bursts, the concept of an “effective thermal partition” is used, together with the normal transmittance, such as given in Fig. 7.98, for the existing atmospheric conditions. Based upon experimental data, contact surface bursts can be represented fairly well by an effective thermal partition of 0.18. Values of the thermal partition for other surface bursts are shown in Table 7.101 they have been derived by as signing a thermal partition of 0.18 to a contact surface burst and interpolating between that value and the air burst thermal partition values in Table 7.88.
| Thermal Partition | |||||
|---|---|---|---|---|---|
| Total Yield (kilotons) | |||||
| Height of Burst (feet) | 1 | 10 | 100 | 1,000 | 10,000 |
| 20 | 0.19 | * | * | * | * |
| 40 | 0.21 | 0.19 | * | * | * |
| 70 | 0.23 | 0.21 | 0.19 | * | * |
| 100 | 0.26 | 0.22 | 0.20 | * | * |
| 200 | 0.35 | 0.25 | 0.21 | 0.19 | * |
| 400 | ** | 0.33 | 0.25 | 0.21 | 0.19 |
| 700 | ** | ** | 0.28 | 0.24 | 0.21 |
| 1,000 | ** | ** | 0.34 | 0.26 | 0.22 |
| 2,000 | ** | ** | ** | 0.34 | 0.26 |
| 4,000 | ** | ** | ** | ** | 0.33 |
| 7,000 | ** | ** | ** | ** | 0.35 |
VERY-HIGH-ALTITUDE BURSTS
7.102 In the calculation of the thermal radiation exposure at the surface of the earth from very-high-altitude nuclear explosions, two altitude regions must be considered because of the change in the fireball behavior that occurs at altitudes in the vicinity of about 270,000 feet (§ 7.91). At burst heights from roughly 160,000 to 200,000 feet (30 to 38 miles), the thermal energy capable of causing damage at the surface of the earth drops sharply from about 60 percent to about 25 per cent, i.e., from to . As the height of burst is increased above 200,000 feet, the thermal partition remains about 0.25 up to a height of burst of approximately 260,000 feet (49 miles). Since a nearly spherical fireball forms within this latter altitude region, equation (7.93.3) becomes
where is the slant range in kilofeet, and is the yield in kilotons. A linear interpolation of the variation of thermal partition with burst altitude may be performed for bursts between 160,000 feet and 200,000 feet; however, in view of the uncertainties in high-altitude burst phenomenology, it may be desirable to use the high (0.60) or the low (0.25) value throughout this burst altitude region, depending on the degree of conservatism desired.
7.103 At burst altitudes of roughly 270,000 feet and above, the thermal radiation is emitted from the thick X-ray pancake at a mean altitude of about 270,000 feet, essentially independent of the actual height of burst (§ 7.91). In order to use the equations in § 7.96 to calculate radiant exposures at various distances from the burst, the approximation is made of replacing the disklike radiating region by an equivalent source point defined in the following manner. If the distance from ground zero to the target position where is to be calculated is less than the height of burst, , the source may be regarded as being located at the closest point on a circle with the median radius at an altitude of 270,000 feet; this is indicated by the point S in Fig. 7.103. Hence, for the target point X, the appropriate slant range is given approximately by
with and in kilofeet. This expression holds even when is greater than ; although the quantity in the square brackets is then negative, the square is positive. The slant range, , for ground zero is obtained by setting in equation (7.103. l) equal to zero; thus,
If the distance is greater than the height of burst, the equivalent point source may be taken to be approximately at the center of the radiating disk at 270,00 feet altitude; then
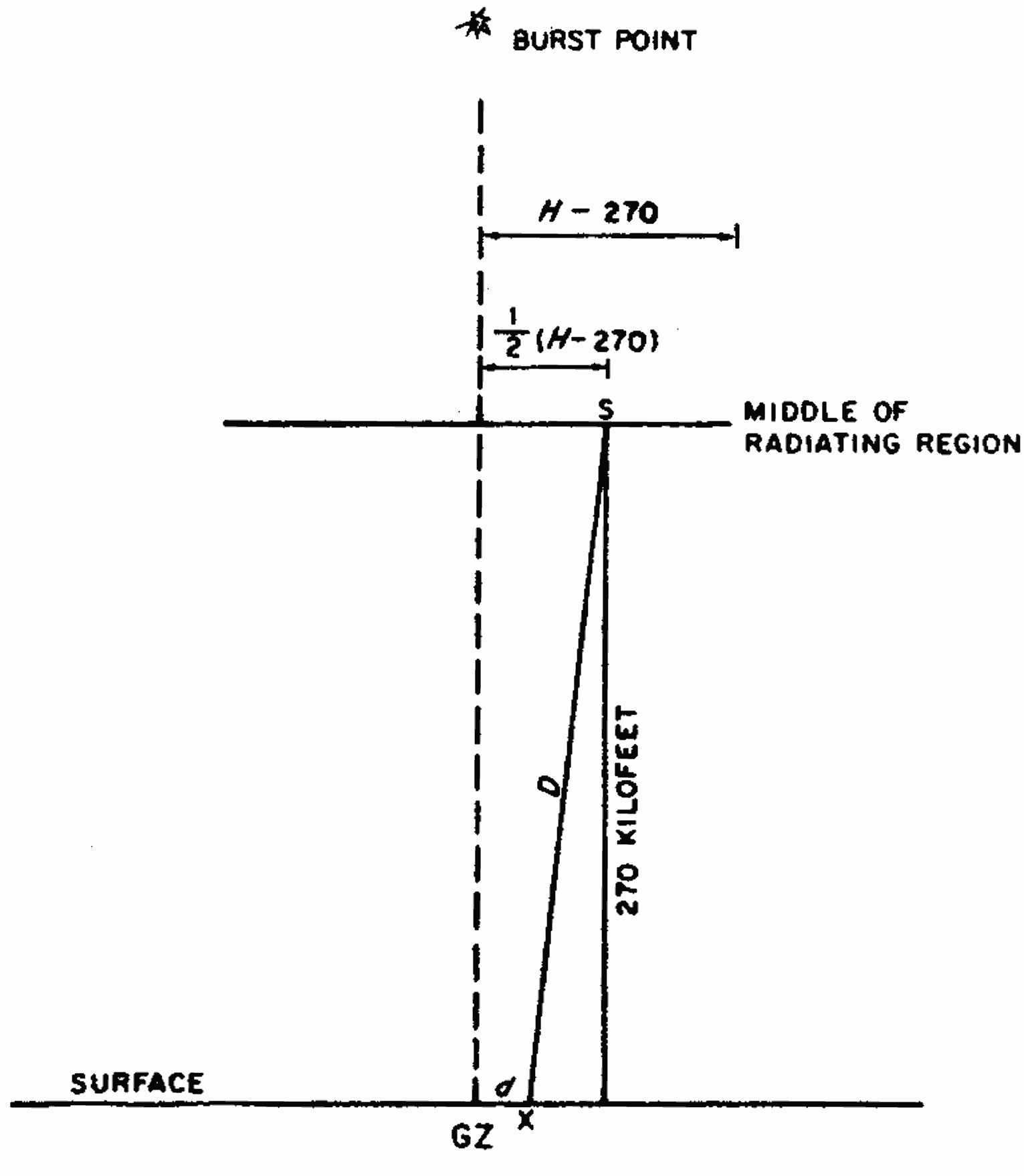
7.104 For the heights of burst under consideration, it is assumed that the fraction 0.8 of the total yield is emitted as X-ray energy and that 0.25 of this energy is absorbed in the radiating disk region. Hence, 0.8 x 0.25 = 0.2 of the total yield is absorbed. For calculating the radiant exposure, the total yield in the equations in § 7.96 is consequently replaced by . Furthermore, the equivalent of the thermal partition is called the “thermal efficiency,” ε, defined as the effective fraction of the absorbed energy that is reradiated. Hence equation (7.96.3), for example, becomes
where in kilofeet is determined in accordance with the conditions described in the preceding paragraph. The values of ε given in Fig. 7.104 as a function of height of burst and yield were obtained by theoretical calculations.6 The transmittance may be estimated from Fig. 7.98 but no serious error would be involved by setting it equal to unity for the large burst heights involved.
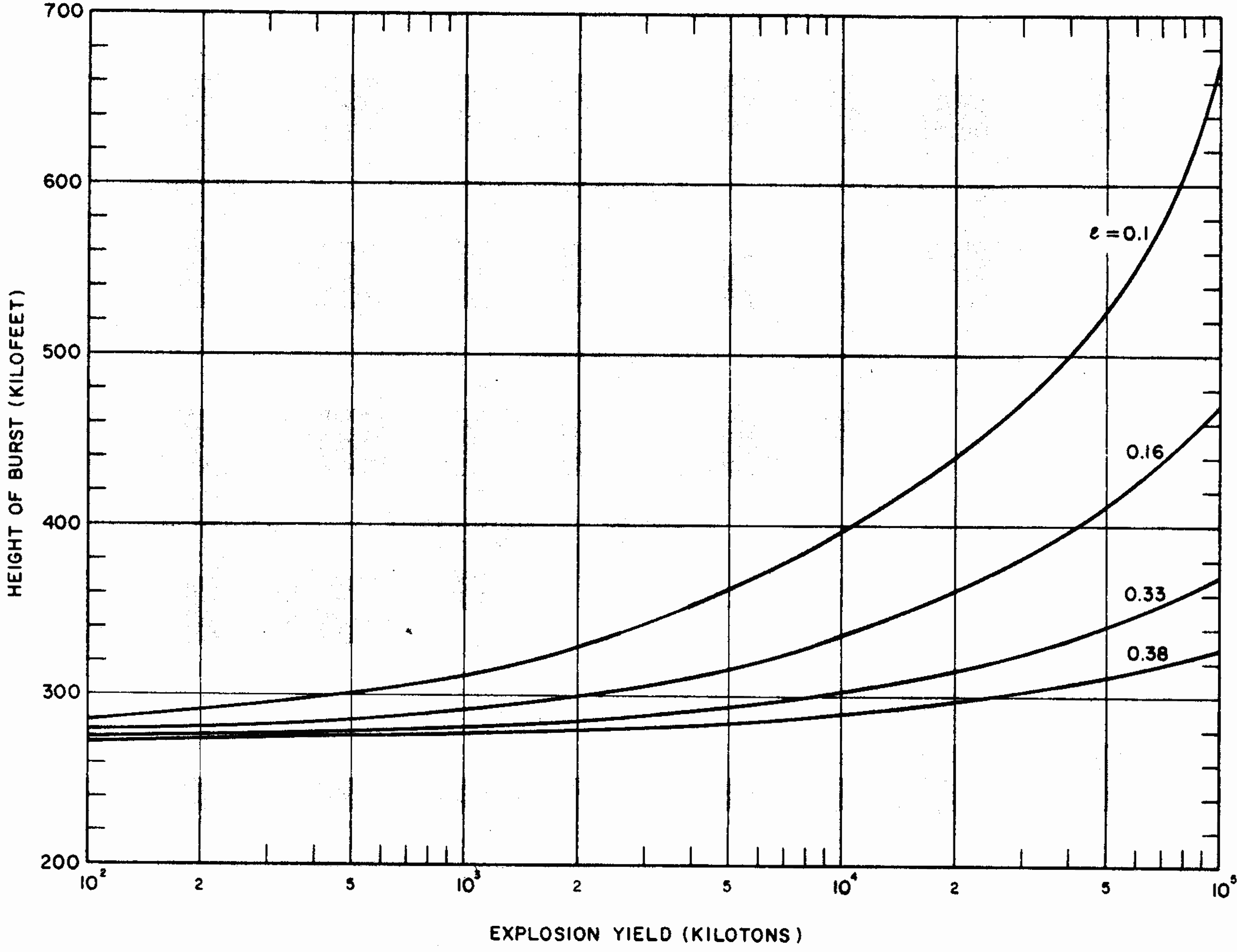
7.105 With the information given above, it is possible to utilize the equations in § 7.96 to calculate the approximate radiant exposure, , for points on the earth’s surface at a given distance, , from ground zero, for a prescribed height of burst, , for explosions of essentially all burst altitudes. If and are specified, the appropriate slant range can be determined. Tables 7.88 and 7.101 and Fig. 7.104 are used to obtain the required thermal partition or thermal efficiency, and the transmittance can be estimated from Fig. 7.98 for the known and . Suppose, however, it is required to reverse the calculations and to find the slant range to a surface target (or the corresponding distance from ground zero for a specified height of burst) at which a particular value of will be attained. The situation is then much more difficult because τ can be estimated only when the slant range or distance from ground zero is known. One approach would be to prepare figures like Fig. 7.42 for several heights of burst and to interpolate among them for any over burst height. Another possibility is to make use of an interation procedure by guessing a value of τ, e.g., τ = 1, to determine a first approximation to . With this value of and the known height of burst, an improved estimate of τ can be obtained from Fig. 7.98. This is then utilized to derive a better approximation to , and so on until convergence is attained.
BIBLIOGRAPHY
- , et al., “Blast Wave,” University of California, Los Alamos Scientific Laboratory, March 1958, LA–2000.
- , “Review of Nuclear Weapons Effects,” Ann. Rev. Nuclear Sci., 18, 153 (1968).
- , et al., “Prediction of Fire Spread Following Nuclear Explosions,” Pacific Southwest Forest and Range Experiment Station, Berkeley, California, 1963, U.S. Forest Service Paper PSW–5.
- , “Transmissivity of the Atmosphere for Thermal Radiation from Nuclear Weapons,” U.S. Naval Radiological Laboratory, August 1966, USNRDL–TR–1060.
- “Effects of Air Blast on Urban Fires,” URS Research Co., Burlingame, California, December 1970, OCD Work Unit 25341.
- , “Reflection of Point Source Radiation from a Lambert Plane onto a Plane Receiver,” Air Force Cambridge Research Center, TR–57–253, Library of Congress, Washington, D.C., 1957.
- , “Studies on Thermal Radiation,” Cornell University Medical College, PB 154–803, Library of Congress, Washington, D.C., 1952.
- , “Thermal Ignition and Response of Materials,” Office of Civil Defense and Mobilization, 1957, WT–1198.
- , “The Role of Fire in Nuclear Warfare: An Interpretative Review of the Current Technology for Evaluating the Incendiary Consequences of the Strategic and Tactical Uses of Nuclear Weapons,” URS Research Co., San Mateo, California, August 1974, DNA 2692F.
- , “Vision Through the Atmosphere,” University of Toronto Press, 1958.
- , “Radiative Transfer from Nuclear Detonations Above 50-Km Altitude,” Fire Research Abstracts and Reviews, 6, 99 (1964), National Academy of Sciences–National Research Council.
- , “Damage to Conventional and Special Types of Residences Exposed to Nuclear Effects,” Office of Civil Defense and Mobilization, March 1961, WT–1194.
- , “Heal Transfer in Thermal Radiation Absorbing and Scattering Material,” Argonne National Laboratory, May 1960, ANL 6170.
- , “Evaluation of the Nuclear Fire Threat to Urban Areas,” Stanford Research Institute, Menlo Park, California, September 1973, SRI PYU– 11150_
*These documents may be purchased from the National Technical Information Service, Department of Commerce, Springfield, Virginia, 22161.
**These documents may be obtained from the Library of Congress, Washington, D.C. 20402.
ENDNOTES
- 1 It is known, from theoretical studies and experimental measurements, that the wavelength corresponding to the maximum energy density of radiation from an ideal (or “black body”) radiator, to which the nuclear fireball is a good approximation, decreases with increasing temperature of the radiation. At temperatures above 7,500°K (I 3,000°F), this maximum lies in the ultraviolet and X-ray regions of the spectrum (§ 7.78). [ref. § 7.23] X
- 2 The thermal radiation energy incident on the front of the house referred to in § 7.28 was about 25 cal/cm2. [ref. § 7.38] X
- 3 The effects of thermal radiations on people in Japan are described in Chapter XII. [ref. THERMAL EFFECTS ON MATERIALS IN JAPAN] X
- 4 The remaining sections of this chapter may be omitted without loss of continuity. [ref. TECHNICAL ASPECTS OF THERMAL RADIATION] X
- 5 Scattered radiation does not cause permanent damage to the retina of the eye. Hence, to determine the effective radiant exposure in this connection equation (7.94.1) should be used; κ is about 0.03 km-1 for a visibility of 80 km (50 miles), 0.1 km-1 for 40 km (25 miles) and 0.2 km-1 for 20 km (12.4 miles). Scattered radiation can, however, contribute to flashblindness, resulting from the dazzling effect of bright light (§ 12.83). [ref. § 7.95] X
- 6The calculations are actually for the fraction of the absorbed X-ray energy reradiated within 10 seconds; for estimating effects on the ground, the subsequent reradiation can be neglected. [ref. § 7.104] X